Question: We still consider the binary classification problem on X, and we denote fn: F(X,Y) a classifier. We say that it is universally consistent in

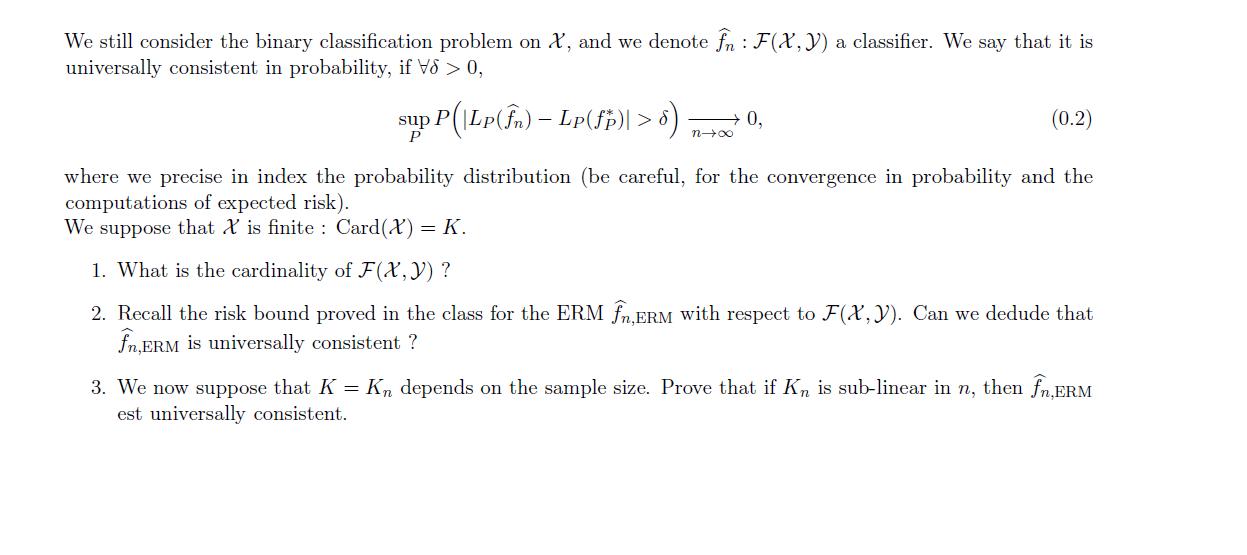
We still consider the binary classification problem on X, and we denote

We still consider the binary classification problem on X, and we denote fn: F(X,Y) a classifier. We say that it is universally consistent in probability, if V > 0, sup P(|Lp(n) Lp(fp)| > 6) na 0, (0.2) where we precise in index the probability distribution (be careful, for the convergence in probability and the computations of expected risk). We suppose that is finite: Card(X) = K. 1. What is the cardinality of F(X,Y)? 2. Recall the risk bound proved in the class for the ERM fn,ERM with respect to F(X,Y). Can we dedude that fn,ERM is universally consistent ? 3. We now suppose that K = Kn depends on the sample size. Prove that if K is sub-linear in n, then fn,ERM est universally consistent.

Step by Step Solution

★★★★★

3.47 Rating (160 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

A 1 defects size B D E 88888888888888888888888888 2 21 3 24 4 16 5 12 6 15 7 5 8 28 9 20 10 31 11 25 12 20 13 24 14 16 15 19 16 10 17 17 18 13 19 22 20 18 21 39 22 30 23 24 24 16 25 19 26 17 27 15...

1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 A B Total assets Sales Debt-equity ratio Return on equity D $ $ Net income 2,604 5,783 0.75 E 11% F Y3K, Inc., has sales of $5,783, total assets of...

1 2 3 4 5 6 7 8 9 10 Call price 11 A B Raider stock price 12 at option expiration, ST 13 14 15 16 17 18 19 20 21 22 23 0 10 20 30 40 50 60 70 80 90 100 C What is their combined profit at any S, and...

Consider a binary classification problem with two-dimensional features, i.e., K = 2, p = 2. Class 1 has multivariate Gaussian distribution N (1 , ), and class 2 has multivariate Gaussian distribution...

Consider a binary classification problem over classes 0 and 1, where the data distribution is the following: . _ 1 with probability 1/2 x- Uniform([1, 2]) if yi = 1 31, 0 with probability 1/2, 1...

Consider a binary classification problem with the following set of attributes and attribute values: ¢ Air Conditioner = {Working, Broken} ¢ Engine = {Good, Bad} ¢ Mileage = {High,...

Consider a binary classification problem where the response $Y$ takes values in $\{-1,1\}$. Show that optimal prediction function for the hinge loss \(\operatorname{Loss}(y, \tilde{y})=(1-y...

Consider a binary classification problem with an ensemble learning algorithm that uses simple majority voting among K learned hypotheses. Suppose that each hypothesis has error E and that the errors...

Consider a binary classification problem whereXRpandY {0,1}. For a fixedxRp, suppose that Pr(Y= 1|X=x) =pfor somep[0,1]. Consider a prediction problem where there is a lossLFN>0 associated with...

Consider a binary classification problem in which each observation xn is known to belong to one of two classes, corresponding to t = 0 and t = 1, and suppose that the procedure for collecting...

Kevin Smith, a plant manager at XYZ Corp must choose between two new machines with unequal lives. Both machines can do the job but they have different operating costs and will last for different time...

Consider a consumer with a utility function u (x1, x2) = Vx + x2 .lf the prices for goods 1 and 2 are 2 and 1 respectively, and income is 20, what is the consumer's optimal consumption of good 1?

Convert the point ( r , , z ) = ( 2 , 5 6 , 3 ) t o Cartesian coordinates. Give answers either a s expressions, o r decimals t o a t least one decimal place. ( x , y , z ) = 1

The Pattia Winery is one of the finest wineries in the country. One of its famous produces is a red wine called Old Vines. Recently, management has become concerned about the increasing cost of...

On January 1, 20--, a depreciable asset was acquired for $5,760. The asset has an estimated useful life of four years (48 months) and no salvage value . Use the straight-line depreciation method to...

When is a hybrid costing system appropriate in a manufacturing setting?

Prince Electronics, a manufacturer of consumer electronic goods, has five distribution centers in different regions of the country. For one of its products, a high- speed modem priced at $350 per...

After completing Steps 1-4 of the process costing procedure, Peterson Corporation arrived at the following equivalent units and costs per equivalent unit for its final production department for the...

What is the most important constitutional duty for the comptroller of public accounts? Group of answer choices Certifying the financial condition of the state at the close of each fiscal year Acting...

The feature of arbitrage pricing theory (APT) that offers the greatest potential advantage over the simple CAPM is the: a. Identification of anticipated changes in production, inflation, and term...

According to the APT, if the risk-free rate is 4%, what should be McCrackens estimate of the expected return of Orbs High Growth Fund? Orb Trust (Orb) has historically leaned toward a passive...

Another committee member suggested that, rather than evaluate each managed portfolio independently of other portfolios, it might be better to consider the effects of a change in the Hennessy...

2. What is the difference between temporal summation and spatial summationpg99

2. A pinch on an animals right hind foot excites a sensory neuron that excites an interneuron that excites the motor neurons to the flexor muscles of that leg. The interneuron also inhibits the motor...