Question
Attach workings please. Please use R studio, Thank you. 2. The probability of a student passing statistics is known to be 0.41; and the probability
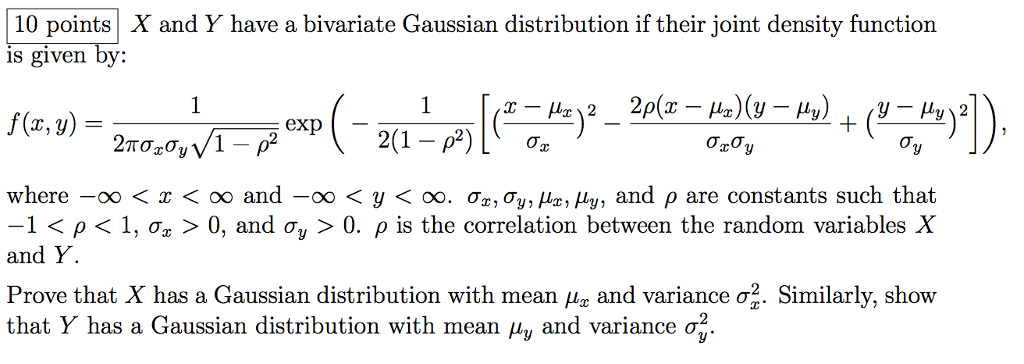
Attach workings please.
Please use R studio, Thank you.
2. The probability of a student passing statistics is known to be 0.41; and the probability of a student passing chemistry is known to be 0.55. If the probability of passing both is known to be 0.35, calculate:
(a) the probability of passing at least one of statistics and chemistry
(b) the probability of a student passing chemistry, given that they passed statistics
(c) Are passing chemistry and statistics independent? Justify
(d) (harder) a group of 33 randomly selected students attend a special seminar on study skills. Of these 33, only 7 fail both. State a sensible null hypothesis, test it, and interpret
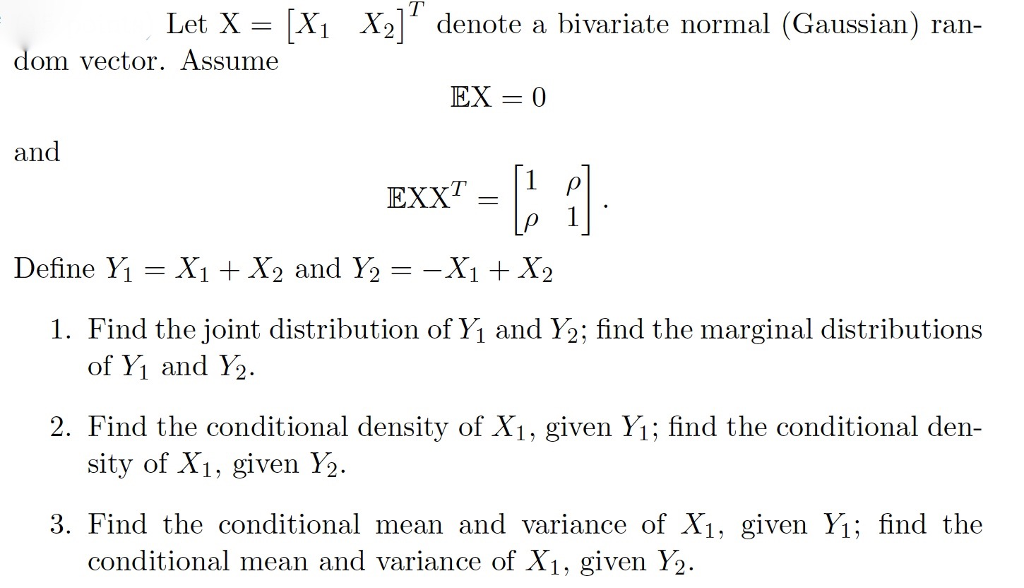

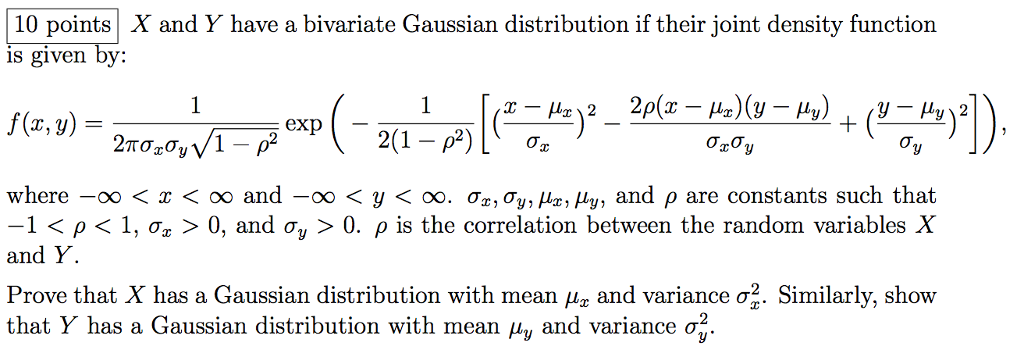
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


