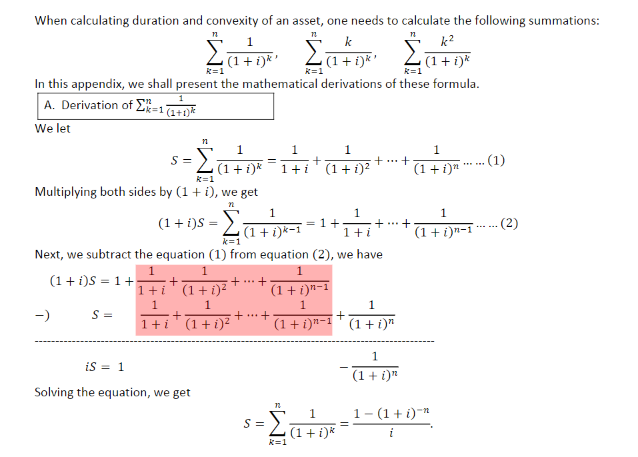
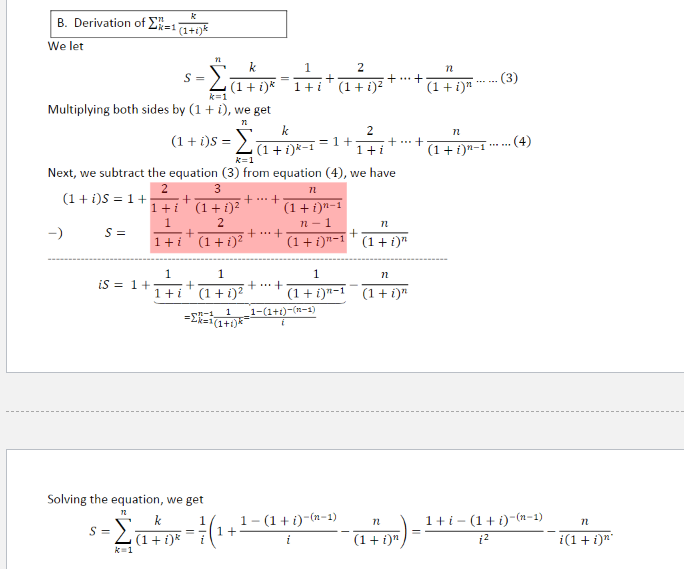
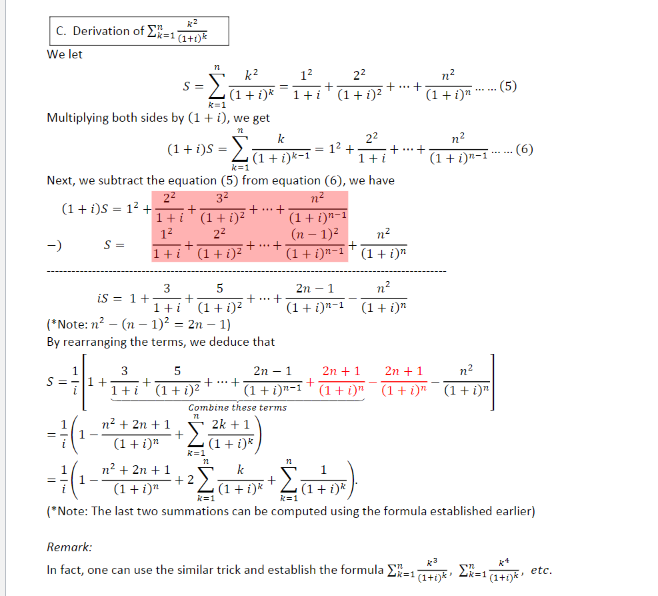
Bonus Problem 1 (Optional, 20 marks) We consider a nT -year coupon bond which pays coupons every T years. The bond has face value F and the coupon rate over a coupon period is r (i.e. the amount of each coupon payment is rF). The annual effective yield rate of the bond isi. Question Derive the formula for the Macaulay duration and Modified convexity of the bond (with respect to annual effective interest rate i). Express your answer in terms of n. 7,r, Fandi. (Hint: The following formula will be useful. 72 = = 1 1-(1+i)- (1+i)* i 1 1-(1+1)-(n-1) n 1+i-(1 + i)-(n-1) 1+ (1+i)* i (1 + i)" ka n2 + 2n + 1 1 1 +2 + (1 + i)* (1+i)n (1 + ) (1 + )k k=1 The derivation of these formula can be found in the appendix A) n i(1 + i)" i 17) 12 = =;(: +5) k=1 k1 : + E=1 =1 E=1 When calculating duration and convexity of an asset, one needs to calculate the following summations: 1 k k2 1 + ' (1+ In this appendix, we shall present the mathematical derivations of these formula. A. Derivation of EX=1 (1+0% We let 1 1 1 1 S = + +...+ (1 + i)2 1+i (1) (1 + 1) Multiplying both sides by (1 + i), we get 1 (1 + i)s - . = 1 + +...+ (2) (1 + i)k-1 1+i (1 + in-1 Next, we subtract the equation (1) from equation (2), we have 1 (1+i)S = 1+. + + + 1+i' (1 + i) (1 + i)n-1 1 1 -) S = + ... + 1+i (1 + i)2 (1 + 1)1-1 (1+i)" -1 +1) TE =1 1 1 k=1 1 1 1 + iS = 1 Solving the equation, we get 1 (1 + 1) 72 1-(1 + i)** S = 1 (1+i)* ke1 B. Derivation of ER=1 (1+0)* n n Eu+i)* = (3) 72 n We let k 1 2 n S = + +++ 1+1 (1 + i)2 (1 + i)" k=1 Multiplying both sides by (1 + i), we get k 2 = 1+ + + 1+1 (1+i)n-1 k=1 Next, we subtract the equation (3) from equation (4), we have 2 3 (1 + i)S = 1 + + + ... + 1+1 (1 + 1)2 (1+i)n-1 1 2 n - 1 72 -) S = + + **+ + 1+i(1+i)2 (1 + i)n-1 *(1 + i)" (1 + i)5 = Eatok-1 = (4) 71 1 1 is = 1+ + 1+i" (1 + i) 1 n +++ (1+i)n-1 (1 + i)" 1-(1+1)-(n-1) (1+0)* Solving the equation, we get k 1 S (1 + i)* 7 n - Zo 1-(1 + i)-(n-1) + i 1+ i-(1 + i)(n-1) i2 (1 + i) i(1+i) k R2 C. Derivation of Ex=1 (1+0) n .(5) ) REF R=1 We let k2 12 22 n2 S = + +...+ (1 + i)* 1+i (1 + i)2 (1 + i)" Multiplying both sides by (1 + i), we get k 22 n2 12 + + ... + (1 + i)k-1 1+1 (1 + i)n-1 k=1 Next, we subtract the equation (5) from equation (6), we have 22 32 n2 (1 + i)S = 12 + + + ... + 1+ i (1 + i) (1+i)n-1 12 22 (n 1)2 -) + + + + 1+i (1 + i)2 (1 + i)"-1 (1 + i)" (1 + i)s = 24+ .. (6) S= n2 (1 + i)" 3 5 2n-1 is = 1+ + + + 1+i(1 + i) (1 + i)n-1 (*Note: n - (n-1)2 = 2n-1) By rearranging the terms, we deduce that 1 71 = 3 5 2n-1 2n + 1 2n + 1 n2 S= 1+ + + ... + + 1+i*(1 + i)2 (1 + i)n-1 *(1+in (1+i)n (1 + i)" Combine these terms n2 + 2n +1 2k + 1 + (1 + i)" (1 + i)* n? + 2n +1 k 1 + 2 + (1 + i)" (1 + i)* (1 + i)* (* Note: The last two summations can be computed using the formula established earlier) R=1 - 11 =(1- +33) R=1 R=1 Remark: In fact, one can use the similar trick and establish the formula EX=11+5)*: EX=1(1+00** R3 etc. Bonus Problem 1 (Optional, 20 marks) We consider a nT -year coupon bond which pays coupons every T years. The bond has face value F and the coupon rate over a coupon period is r (i.e. the amount of each coupon payment is rF). The annual effective yield rate of the bond isi. Question Derive the formula for the Macaulay duration and Modified convexity of the bond (with respect to annual effective interest rate i). Express your answer in terms of n. 7,r, Fandi. (Hint: The following formula will be useful. 72 = = 1 1-(1+i)- (1+i)* i 1 1-(1+1)-(n-1) n 1+i-(1 + i)-(n-1) 1+ (1+i)* i (1 + i)" ka n2 + 2n + 1 1 1 +2 + (1 + i)* (1+i)n (1 + ) (1 + )k k=1 The derivation of these formula can be found in the appendix A) n i(1 + i)" i 17) 12 = =;(: +5) k=1 k1 : + E=1 =1 E=1 When calculating duration and convexity of an asset, one needs to calculate the following summations: 1 k k2 1 + ' (1+ In this appendix, we shall present the mathematical derivations of these formula. A. Derivation of EX=1 (1+0% We let 1 1 1 1 S = + +...+ (1 + i)2 1+i (1) (1 + 1) Multiplying both sides by (1 + i), we get 1 (1 + i)s - . = 1 + +...+ (2) (1 + i)k-1 1+i (1 + in-1 Next, we subtract the equation (1) from equation (2), we have 1 (1+i)S = 1+. + + + 1+i' (1 + i) (1 + i)n-1 1 1 -) S = + ... + 1+i (1 + i)2 (1 + 1)1-1 (1+i)" -1 +1) TE =1 1 1 k=1 1 1 1 + iS = 1 Solving the equation, we get 1 (1 + 1) 72 1-(1 + i)** S = 1 (1+i)* ke1 B. Derivation of ER=1 (1+0)* n n Eu+i)* = (3) 72 n We let k 1 2 n S = + +++ 1+1 (1 + i)2 (1 + i)" k=1 Multiplying both sides by (1 + i), we get k 2 = 1+ + + 1+1 (1+i)n-1 k=1 Next, we subtract the equation (3) from equation (4), we have 2 3 (1 + i)S = 1 + + + ... + 1+1 (1 + 1)2 (1+i)n-1 1 2 n - 1 72 -) S = + + **+ + 1+i(1+i)2 (1 + i)n-1 *(1 + i)" (1 + i)5 = Eatok-1 = (4) 71 1 1 is = 1+ + 1+i" (1 + i) 1 n +++ (1+i)n-1 (1 + i)" 1-(1+1)-(n-1) (1+0)* Solving the equation, we get k 1 S (1 + i)* 7 n - Zo 1-(1 + i)-(n-1) + i 1+ i-(1 + i)(n-1) i2 (1 + i) i(1+i) k R2 C. Derivation of Ex=1 (1+0) n .(5) ) REF R=1 We let k2 12 22 n2 S = + +...+ (1 + i)* 1+i (1 + i)2 (1 + i)" Multiplying both sides by (1 + i), we get k 22 n2 12 + + ... + (1 + i)k-1 1+1 (1 + i)n-1 k=1 Next, we subtract the equation (5) from equation (6), we have 22 32 n2 (1 + i)S = 12 + + + ... + 1+ i (1 + i) (1+i)n-1 12 22 (n 1)2 -) + + + + 1+i (1 + i)2 (1 + i)"-1 (1 + i)" (1 + i)s = 24+ .. (6) S= n2 (1 + i)" 3 5 2n-1 is = 1+ + + + 1+i(1 + i) (1 + i)n-1 (*Note: n - (n-1)2 = 2n-1) By rearranging the terms, we deduce that 1 71 = 3 5 2n-1 2n + 1 2n + 1 n2 S= 1+ + + ... + + 1+i*(1 + i)2 (1 + i)n-1 *(1+in (1+i)n (1 + i)" Combine these terms n2 + 2n +1 2k + 1 + (1 + i)" (1 + i)* n? + 2n +1 k 1 + 2 + (1 + i)" (1 + i)* (1 + i)* (* Note: The last two summations can be computed using the formula established earlier) R=1 - 11 =(1- +33) R=1 R=1 Remark: In fact, one can use the similar trick and establish the formula EX=11+5)*: EX=1(1+00** R3 etc