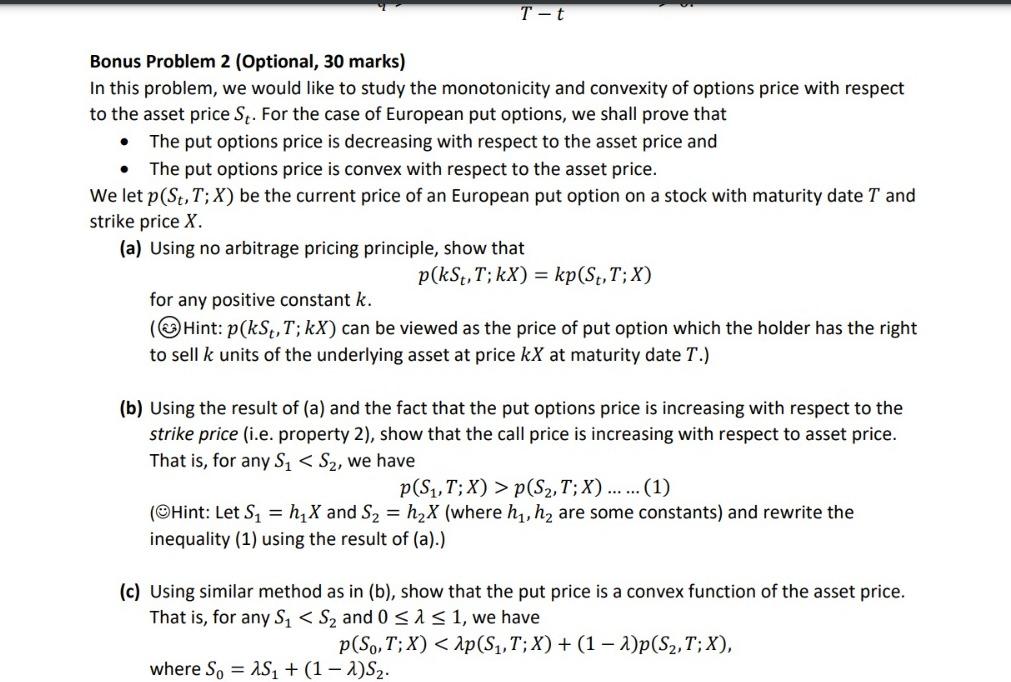Bonus Problem 2 (Optional, 30 marks) In this problem, we would like to study the monotonicity and convexity of options price with respect to the asset price . For the case of European put options, we shall prove that The put options price is decreasing with respect to the asset price and The put options price is convex with respect to the asset price. We let ( , ; ) be the current price of an European put option on a stock with maturity date and strike price . (a) Using no arbitrage pricing principle, show that ( , ; ) = ( , ; ) for any positive constant . (Hint: ( , ; ) can be viewed as the price of put option which the holder has the right to sell units of the underlying asset at price at maturity date .) (b) Using the result of (a) and the fact that the put options price is increasing with respect to the strike price (i.e. property 2), show that the call price is increasing with respect to asset price. That is, for any 1 (2, ; ) (1) (Hint: Let 1 = 1 and 2 = 2 (where 1, 2 are some constants) and rewrite the inequality (1) using the result of (a).) (c) Using similar method as in (b), show that the put price is a convex function of the asset price. That is, for any 1 T-t . . Bonus Problem 2 (Optional, 30 marks) In this problem, we would like to study the monotonicity and convexity of options price with respect to the asset price S. For the case of European put options, we shall prove that The put options price is decreasing with respect to the asset price and The put options price is convex with respect to the asset price. We let p(St,T;X) be the current price of an European put option on a stock with maturity date T and strike price X. (a) Using no arbitrage pricing principle, show that p(kSt, T;kx) = kp(St,T;X) for any positive constant k. (Hint: p(kS,,T;kx) can be viewed as the price of put option which the holder has the right to sell k units of the underlying asset price kX at maturity date T.) (b) Using the result of (a) and the fact that the put options price is increasing with respect to the strike price (i.e. property 2), show that the call price is increasing with respect to asset price. That is, for any S, p(S2,T;X) ...... (1) (Hint: Let S = h X and S2 = h2X (where hy, hy are some constants) and rewrite the inequality (1) using the result of (a).) (c) Using similar method as in (b), show that the put price is a convex function of the asset price. That is, for any S, p(S2,T;X) ...... (1) (Hint: Let S = h X and S2 = h2X (where hy, hy are some constants) and rewrite the inequality (1) using the result of (a).) (c) Using similar method as in (b), show that the put price is a convex function of the asset price. That is, for any S,