Buck's is a small campus shop that offers two house-made protein bars: Green Bar and Super Bar. Each bar requires protein and wheat.

- Buck's has 50 cups of protein and 48 cups of wheat available.

- A pack of Green Bar uses 5 cups of protein and 6 cups of wheat.

- A pack of Super Bar uses 10 cups of protein and 8 cups of wheat.

- Green Bar has a profit of $90 per pack; Super Bar has a profit of $120 per pack.

- Use Solver to determine the optimal production of Green Bar and Super Bar packs that maximizes Buck's profit. Assume that everything produced will be sold.

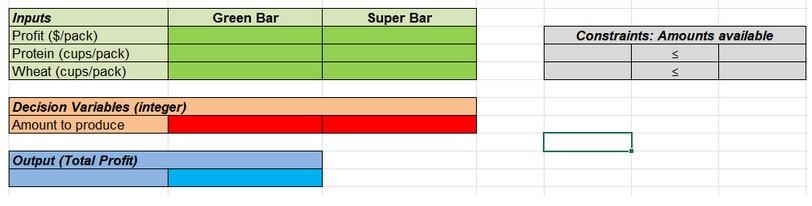
Please replicate the above image/template in excel (colors and formatting don't matter) and paste a picture of the Solver window you used to solve for "Total Profit" (the solution the question is after). For the "constraints box" you can switch the sign if it is more convenient.

\begin{tabular}{|l|c|c|} \hline Inputs & Green Bar & Super Bar \\ \hline Profit ($/ pack) & & \\ \hline Protein (cups/pack) & & \\ \hline Wheat (cups/pack) & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|} \hline \multicolumn{3}{|c|}{ Constraints: Amounts available } \\ \hline & & \\ \hline & & \\ \hline \end{tabular} Decision Variables (integer) Amount to produce Output (Total Profit)