Case 3: 3 2 1

Matrix 3:

Bag size

	0kg	1kg	2kg	3kg	4kg	5kg
0 (no item)	0	0	0	0	0	0
3 (item3)	0	A11	B11	C11	D11	E11
2 (item3/2)	0	F11	G11	H11	I11	J11
1 (item3/2/1)	0	K11	L11	M1	N11	O11

A11: show the math calculation

B11:

O11:

Table 3 (steal or not):

Bag size

	0kg	1kg	2kg	3kg	4kg	5kg
0 (no item)	0	0	0	0	0	0
3 (item3)	0	A12	B12	C12	D12	E12
2 (item3/2)	0	F12	G12	H12	I12	J12
1 (item3/2/1)	0	K12	L12	M12	N12	O12

Analysis for Case 3 (3, 2, 1):

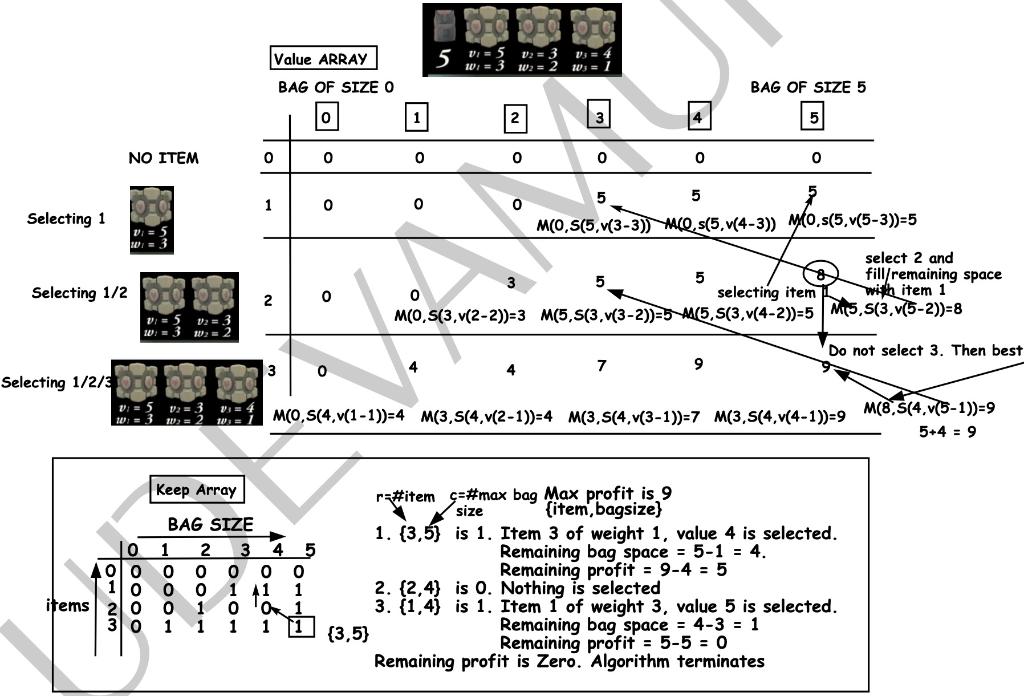
0/1 Knapsack problem: dynamic programming There are 3 items in your house. The thief will come to your house. His maximum bag size is 5kg. The thief wants to maximize the profit, but he can not take more than 5kg.For each item, he can steal (1) or not steal (0). Item1: value =$5, weight =3kg Item2: value =$3, weight =2kg Item3: value =$4, weight =1kg No coding is needed. 1. Please fill in the matrix of bag size (column) and item (row) for the case of 3,2,1 And show the math calculation process for each cell in the matrix (bag size, item) 2. Also fill in the table of steal or not steal 3. show the traverse process in the matrix and the analysis for the optimal solution. The optimal solution is that the thief steals item 1 and item 3 with profit of 9 and weight of 4kg The solution for each case should look like this