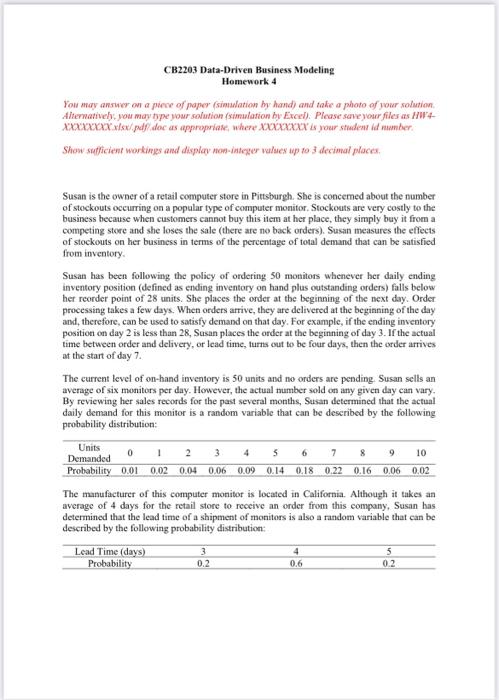
CB2203 Data-Driven Business Modeling

CB2203 Data-Driven Business Modeling Homework 4 You may answer on a pieve of poper (simulation by hand) and take a photo of your solution. Aherwatively, you may tope your solution (ximulation by Exced). Please save your files as lint 4 Show sutficient workings and display non-integer velues up to 3 decimal places. Susan is the owner of a retail computer store in Pittsburgh. She is concemed about the number of stockouts occurring on a popular type of computer monitor. Stockouts are very costly to the business because when customers cannot buy this item at her place, they simply buy it from a competing store and she loses the sale (there are no back orders). Susan measures the effects of stockouts on her business in terms of the pereentage of total demand that can be satisfied from inventory. Susan has been following the policy of ordering 50 monitors whenever her daily ending inventory position (defined as ending inventory on hand plus outstanding orders) falls below her reorder point of 28 units. She places the order at the beginning of the next day. Order processing takes a few days. When orders arrive, they are delivered at the beginning of the day and, therefore, can be used to satisfy demand on that day. For example, if the ending inventory position on day 2 is less than 28 , Susan places the order at the beginning of day 3 . If the actual time between order and delivery, or lead time, turns out to be four days, then the order arrives at the start of day 7 . The current level of on-hand inventory is 50 units and no orders are pending. Susan sells an average of six monitors per day. However, the actual number sold on any given day can vary. By reviewing her sales records for the past several months, Susan determined that the actual daily demand for this monitor is a random variable that can be deseribed by the following probability distribution: The manufacturer of this computer monitor is located in California. Although it takes an average of 4 days for the retail store to receive an order from this company, Susan has determined that the lead time of a shipment of monitors is also a random variable that can be described by the following probability distribution: In parts a to c, you will design a SIMULATION experiment over 15 days (i.e. 15 trials). The following random numbers from 0099 have already been generated List I - Random numbers to determine the units demanded daily. Start at the top of the list. There mav be more random numbers than you need. List 2 - Random numbers to determine the lead time (days) of shipment. Start at the top of the list. There may be more random numbers than you need. a) Using simulation (by hand or by Excel) and the first list of random numbers, num a simulation with 15 trials/days to determine daily demand for the monitors. Start from the TOP of random number List 1. (Start random number assignment from 01.) What are the average quantities of demand for this computer monitor? (42 marks) b) Based on the results of part (a), calculate the following variables for day I: 1) Beginning-day inventory 2) demand satisfied by Susan 3) ending-day iaventory 4) Inventory Position (defined as cnding imventory on hand plus outstanding asders) 5) Order decision (whether Susan should make new orders at the end of the day) (20) marks) c) Based on the results of part (a) and (b), simulate the kead time shipments of Susan's orders, if needed. Start from the TOP of random number List 2. (Start random number assignment from 01 .) What is the average beginning-day inventory of the simulated 15 days? (38 marks) CB2203 Data-Driven Business Modeling Homework 4 You may answer on a pieve of poper (simulation by hand) and take a photo of your solution. Aherwatively, you may tope your solution (ximulation by Exced). Please save your files as lint 4 Show sutficient workings and display non-integer velues up to 3 decimal places. Susan is the owner of a retail computer store in Pittsburgh. She is concemed about the number of stockouts occurring on a popular type of computer monitor. Stockouts are very costly to the business because when customers cannot buy this item at her place, they simply buy it from a competing store and she loses the sale (there are no back orders). Susan measures the effects of stockouts on her business in terms of the pereentage of total demand that can be satisfied from inventory. Susan has been following the policy of ordering 50 monitors whenever her daily ending inventory position (defined as ending inventory on hand plus outstanding orders) falls below her reorder point of 28 units. She places the order at the beginning of the next day. Order processing takes a few days. When orders arrive, they are delivered at the beginning of the day and, therefore, can be used to satisfy demand on that day. For example, if the ending inventory position on day 2 is less than 28 , Susan places the order at the beginning of day 3 . If the actual time between order and delivery, or lead time, turns out to be four days, then the order arrives at the start of day 7 . The current level of on-hand inventory is 50 units and no orders are pending. Susan sells an average of six monitors per day. However, the actual number sold on any given day can vary. By reviewing her sales records for the past several months, Susan determined that the actual daily demand for this monitor is a random variable that can be deseribed by the following probability distribution: The manufacturer of this computer monitor is located in California. Although it takes an average of 4 days for the retail store to receive an order from this company, Susan has determined that the lead time of a shipment of monitors is also a random variable that can be described by the following probability distribution: In parts a to c, you will design a SIMULATION experiment over 15 days (i.e. 15 trials). The following random numbers from 0099 have already been generated List I - Random numbers to determine the units demanded daily. Start at the top of the list. There mav be more random numbers than you need. List 2 - Random numbers to determine the lead time (days) of shipment. Start at the top of the list. There may be more random numbers than you need. a) Using simulation (by hand or by Excel) and the first list of random numbers, num a simulation with 15 trials/days to determine daily demand for the monitors. Start from the TOP of random number List 1. (Start random number assignment from 01.) What are the average quantities of demand for this computer monitor? (42 marks) b) Based on the results of part (a), calculate the following variables for day I: 1) Beginning-day inventory 2) demand satisfied by Susan 3) ending-day iaventory 4) Inventory Position (defined as cnding imventory on hand plus outstanding asders) 5) Order decision (whether Susan should make new orders at the end of the day) (20) marks) c) Based on the results of part (a) and (b), simulate the kead time shipments of Susan's orders, if needed. Start from the TOP of random number List 2. (Start random number assignment from 01 .) What is the average beginning-day inventory of the simulated 15 days? (38 marks)