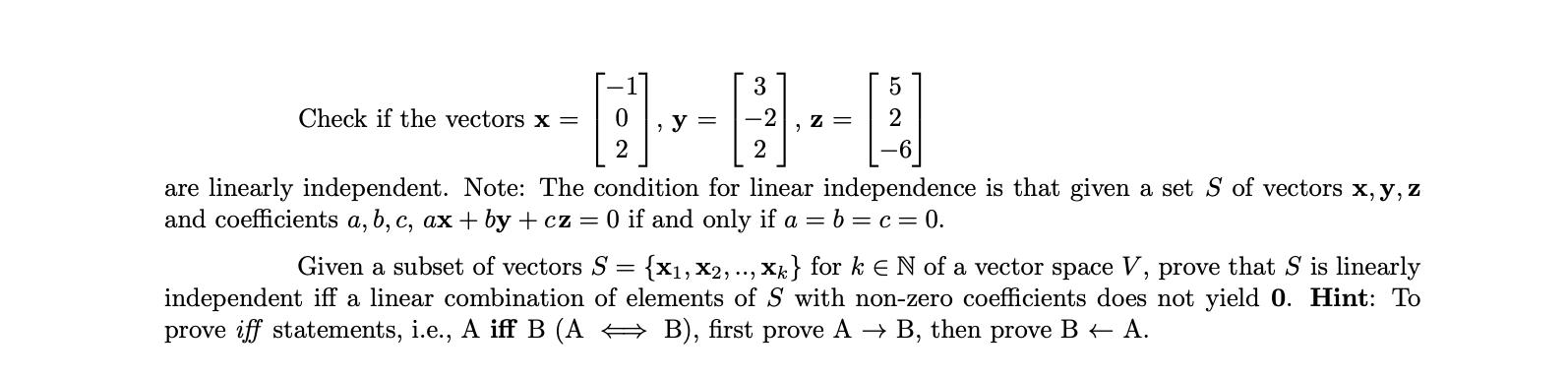
Check if the vectors x = , y = 3 Z = 2 2 2 are linearly independent. Note: The condition for linear independence is that given a set S of vectors x, y, z and coefficients a, b, c, ax+by+cz = 0 if and only if a = b = c = 0. - Given a subset of vectors S {x1,x2,.., xk} for k E N of a vector space V, prove that S is linearly independent iff a linear combination of elements of S with non-zero coefficients does not yield 0. Hint: To prove iff statements, i.e., A iff B (A B), first prove A B, then prove B A.