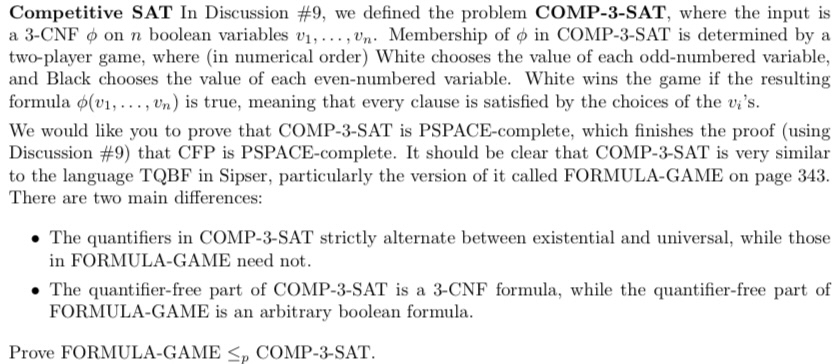
Competitive SAT In Discussion #$9,$ we defined the problem COMP$-3-$SAT, where the input is a $3-$CNF $$ on $n$ boolean variables $v_1,$dots,$v_n.$ Membership of $$ in COMP$-3-$SAT is determined by a two$-$player game, where $($in numerical order$)$ White chooses the value of each odd$-$numbered variable, and Black chooses the value of each even$-$numbered variable. White wins the game if the resulting formula $(v_1,$dots,$v_n)$ is true, meaning that every clause is satisfied by the choices of the $v_i\text{'}$s$.$

We would like you to prove that COMP$-3-$SAT is PSPACE$-$complete, which finishes the proof $($using Discussion #$9)$ that CFP is PSPACE$-$complete. It should be clear that COMP$-3-$SAT is very similar to the language TQBF in Sipser, particularly the version of it called FORMULA$-$GAME on page $343.$ There are two main differences:

The quantifiers in COMP$-3-$SAT strictly alternate between existential and universal, while those in FORMULA$-$GAME need not.

The quantifier$-$free part of COMP$-3-$SAT is a $3-$CNF formula, while the quantifier$-$free part of FORMULA$-$GAME is an arbitrary boolean formula.

Prove FORMULA$-$GAME ${?}_p$ COMP$-3-$SAT.