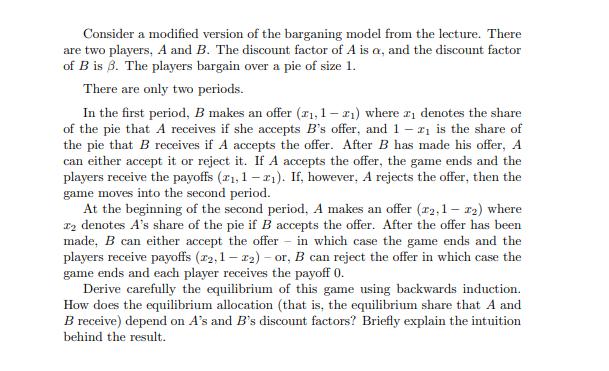
Consider a modified version of the barganing model from the lecture. There are two players, A and B. The discount factor of A is a, and the discount factor of B is 3. The players bargain over a pie of size 1. There are only two periods. In the first period, B makes an offer (2, 1-2) where denotes the share of the pie that A receives if she accepts B's offer, and 1-2 is the share of the pie that B receives if A accepts the offer. After B has made his offer, A can either accept it or reject it. If A accepts the offer, the game ends and the players receive the payoffs (21,1-2). If, however, A rejects the offer, then the game moves into the second period. At the beginning of the second period, A makes an offer (12,1-2) where 1 denotes A's share of the pie if B accepts the offer. After the offer has been made, B can either accept the offer in which case the game ends and the players receive payoffs (22,1-22) - or, B can reject the offer in which case the game ends and each player receives the payoff 0. Derive carefully the equilibrium of this game using backwards induction. How does the equilibrium allocation (that is, the equilibrium share that A and B receive) depend on A's and B's discount factors? Briefly explain the intuition behind the result.