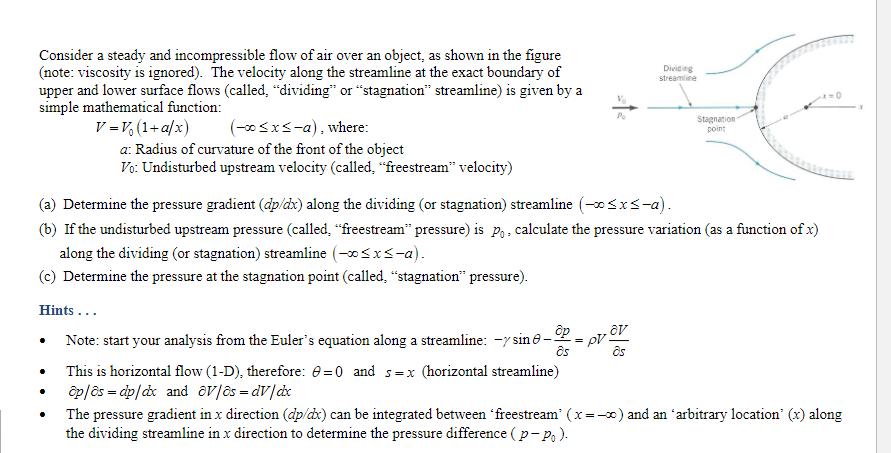
Consider a steady and incompressible flow of air over an object, as shown in the figure (note: viscosity is ignored). The velocity along the streamline at the exact boundary of upper and lower surface flows (called, "dividing" or "stagnation" streamline) is given by a simple mathematical function: . V = V (1+a/x) (-x x -a), where: a: Radius of curvature of the front of the object Vo: Undisturbed upstream velocity (called, "freestream" velocity) . (a) Determine the pressure gradient (dp/dx) along the dividing (or stagnation) streamline (-xx-a). (b) If the undisturbed upstream pressure (called, "freestream" pressure) is po, calculate the pressure variation (as a function of x) along the dividing (or stagnation) streamline (-xx-a). (c) Determine the pressure at the stagnation point (called, "stagnation" pressure). Hints ... Note: start your analysis from the Euler's equation along a streamline: -y sin 8- This is horizontal flow (1-D), therefore: 0 = 0 and s= x (horizontal streamline) op/s = dp/dx and av|s = dv/dx Dividing streamline op , OV Os Os Stagnation point The pressure gradient in x direction (dp/dx) can be integrated between 'freestream (x=-x) and an 'arbitrary location (x) along the dividing streamline in x direction to determine the pressure difference (p-po).