Consider a stock whose price is S₀ and an option on the stock whose current price is v. We suppose that the option lasts for time T and that during the life of the option, the stock price can either move up from S₀ to S₀u or down to a new level S₀d_, where d<1

 

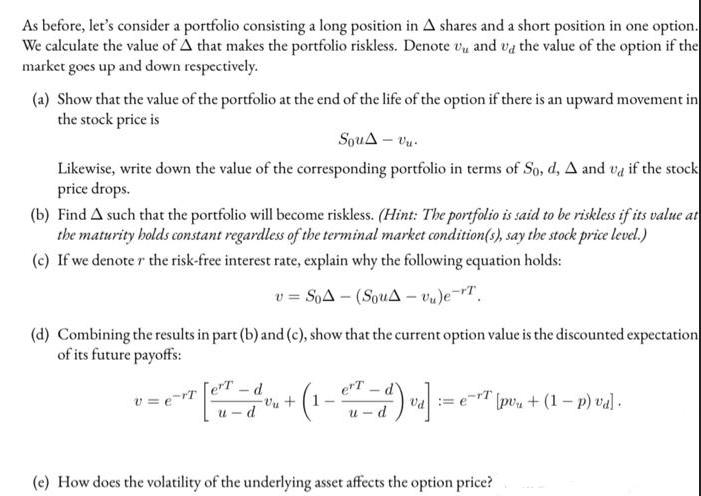
As before, let's consider a portfolio consisting a long position in A shares and a short position in one option. We calculate the value of A that makes the portfolio riskless. Denote U and ua the value of the option if the market goes up and down respectively. (a) Show that the value of the portfolio at the end of the life of the option if there is an upward movement in the stock price is Sous-vu Likewise, write down the value of the corresponding portfolio in terms of So, d, A and va if the stock price drops. (b) Find A such that the portfolio will become riskless. (Hint: The portfolio is said to be riskless if its value at the maturity holds constant regardless of the terminal market condition(s), say the stock price level.) (c) If we denoter the risk-free interest rate, explain why the following equation holds: v=SoA-(SouA-vu)e-T. (d) Combining the results in part (b) and (c), show that the current option value is the discounted expectation of its future payoffs: eT-d u-d ( _e7-4) vd] = u-d Vu + 1 (e) How does the volatility of the underlying asset affects the option price? [pv+(1-p) val.