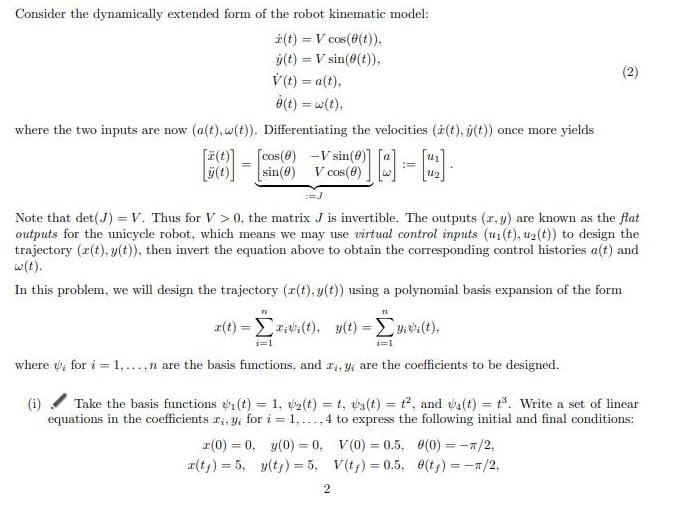
Consider the dynamically extended form of the robot kinematic model: i(t) = V cos(0(t)), (t) = V sin(0(t)), V() = a(t), %3! (2) (t) = w(t), %3! where the two inputs are now (a(t), w(t)). Differentiating the velocities (i(t), (t)) once more yields [#(t)] [cos(0) -V sin(6)] O - sin(0) Vcos(e) U2 Note that det(J) = V. Thus for V > 0, the matrix J is invertible. The outputs (r, y) are known as the flat outputs for the unicycle robot, which means we may use virtual control inputs (u1(t), u2(t)) to design the trajectory (r(t), y(t), then invert the equation above to obtain the corresponding control histories a(t) and w(t). In this problem, we will design the trajectory (r(t), y(t) using a polynomial basis expansion of the form z(t) = Eriv:(t). y(t) = Eu(t), %3D i=1 i=1 where w, for i = 1,...,n are the basis functions, and ri, Yi are the coefficients to be designed. (i) Take the basis functions 1(t) = 1, a(t) = t, ts(t) = t, and va(t) = t. Write a set of linear equations in the coefficients r, Yi for i = 1,...,4 to express the following initial and final conditions: %3D r(0) = 0, y(0) = 0, V(0) = 0.5, 0(0) = -7/2, a(t;) = 5, y(t;) = 5, V(t;) = 0.5, 0(t;) = -T/2, %3D %3D 2 where t = 15. %3D (ii) Why can we not set V(t;) = 0?