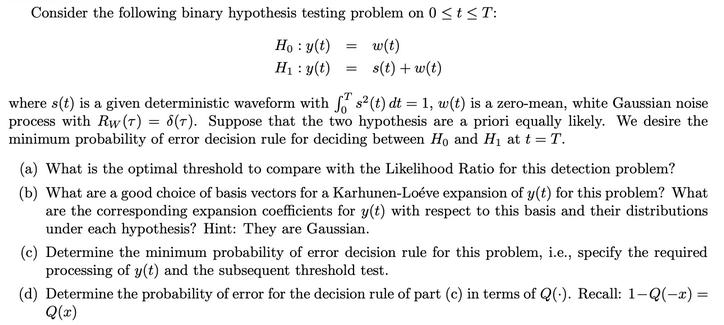
Consider the following binary hypothesis testing problem on 0 tT: Ho:g(t) = w(t) H: y(t) = s(t) + w(t) where s(t) is a given deterministic waveform with fos (t) dt = 1, w(t) is a zero-mean, white Gaussian noise process with Rw (T) = 8(7). Suppose that the two hypothesis are a priori equally likely. We desire the minimum probability of error decision rule for deciding between Ho and H at t = T. (a) What is the optimal threshold to compare with the Likelihood Ratio for this detection problem? (b) What are a good choice of basis vectors for a Karhunen-Love expansion of y(t) for this problem? What are the corresponding expansion coefficients for y(t) with respect to this basis and their distributions under each hypothesis? Hint: They are Gaussian. (c) Determine the minimum probability of error decision rule for this problem, i.e., specify the required processing of y(t) and the subsequent threshold test. (d) Determine the probability of error for the decision rule of part (c) in terms of Q(). Recall: 1-Q(-x) = Q(x)