Question: Consider the following linear programming problem: Max Z = x, + 3x2 subject to: 2x1+ X2 = 0 (C4, C5 ) a. (10 pts)

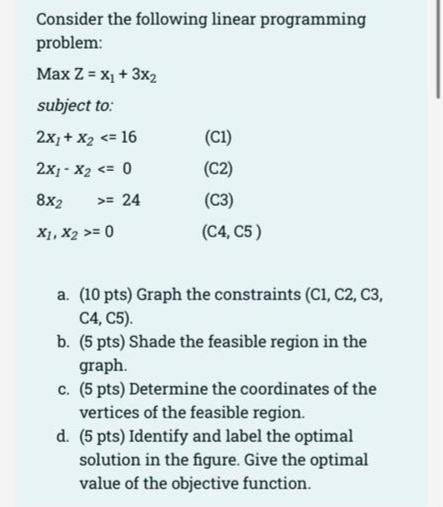
Consider the following linear programming problem: Max Z = x, + 3x2 subject to: 2x1+ X2 = 0 (C4, C5 ) a. (10 pts) Graph the constraints (Cl, C2, C3, C4, C5). b. (5 pts) Shade the feasible region in the graph. c. (5 pts) Determine the coordinates of the vertices of the feasible region. d. (5 pts) Identify and label the optimal solution in the figure. Give the optimal value of the objective function. d. (5 pts) Identify and label the Time left 0:59:33 solution in the figure. Give the optimal value of the objective function. x2 20 18 16 14 12 10 Instructions: 1. Write your answer on a blank paper. 2. Take a picture or scan, and then upload.

Step by Step Solution

★★★★★

3.43 Rating (156 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The Given lpp is max 2 23x2 Subject to 2x4x2 16 21412 ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

Consider the following linear programming problem: Minimize M = 10x + 6y subject to the constraints Determine a point of the feasible set. + z6 4x + 3y s 4 2 0, 20

Consider the following linear programming problem: Maximize profit = 30X1 + 10X2 Subject to: 3X1 + X2 300 X1 + X2 200 X1 100 X2 50 X1 X2 0 X1, X2 0 (a) Solve the problem graphically. (b) Is...

Consider the following nonlinear programming problem: Minimize Z = x41 + 2x22, Subject to x21 + x22 2. (No nonnegativity constraints.) (a) Use geometric analysis to determine whether the feasible...

Consider the following nonlinear programming problem. Maximize Z = 2x21 + 2x2 + 4x3 x23 Subject to 2x1 + x2 + x3 4 and x1 0, x2 0, x3 0. Use dynamic programming to solve this problem.

Consider the following nonlinear programming problem. Maximize Z = x31 + 4x22 + 16x3, Subject to x1 x2 x3 =4 and x1 1, x2 1, x3 1. (a) Solve by dynamic programming when, in addition to the given...

Consider the following convex programming problem: Minimize Z = x4 + x2 4x, Subject to x 2 and x 0.

Consider the following nonlinear programming problem: Minimize Z = 2x1 + x22, subject to x1 + x2 = 10 and x1 0, x2 0. (a) Of the special types of nonlinear programming problems described in Sec....

Consider the following linear programming problem:

Solve the following linear programming model graphically: maximize Z = 1.5x1 + x2 subject to x1 4 x2 6 x1 + x2 5 x1, x2 0

Any written evidence in support of a business transaction is called (A) Journal (B) Ledger (C) Ledger posting (D) Voucher

You give a presentation about technical operations management concepts to an audience that knows very little about the subject. Which McCandless Method concept helps ensure your audience does not...

3. Question 3 Imagine it is currently the first day of 2019. The corporate tax rate is 40 % and the WACC is estimated to be 13 % pa. Suppose also that there is no surplus cash in any year and that...

An analyst for Bloom Ltd, gathered the following information with regards to futures contract: Current spot-market price of R60 Risk-free interest rate of 8.87% per annum The six-month future...

(15 points) On Black Friday, customers arrive at a Gap store at a rate of 100 customers per hour during 6am to 12pm. The store is only able to serve 90 customers per hour. Assume that no more...

Q5. A very simple product {A} consists of a base (B) and a casting (C). The base consists of a plate (P)- and three fasteners (F). There are currently 30 castings and 100 bases on hand. Final...

International cash management systems are more complex than domestic cash management systems because many developing countries still use a cash payments system. some countries rely on electronic...

In your audit of Garza Company, you find that a physical inventory on December 31, 2012, showed merchandise with a cost of $441,000 was on hand at that date. You also discover the following items...

What functions of the firm affect an MRP system? How?

List the disadvantages of simulation.

Shirley Hopkins is developing a program in leadership training for middle-level managers. Shirley has listed a number of activities that must be completed before a training program of this nature...

Perceiving a tomato as consistently red, despite lighting shifts, is an example of shape constancy. perceptual constancy. a binocular cue. continuity.

The snail-shaped tube in the inner ear, where sound waves are converted into neural activity, is called the _______.

Why do you feel a little dizzy immediately after a roller-coaster ride?