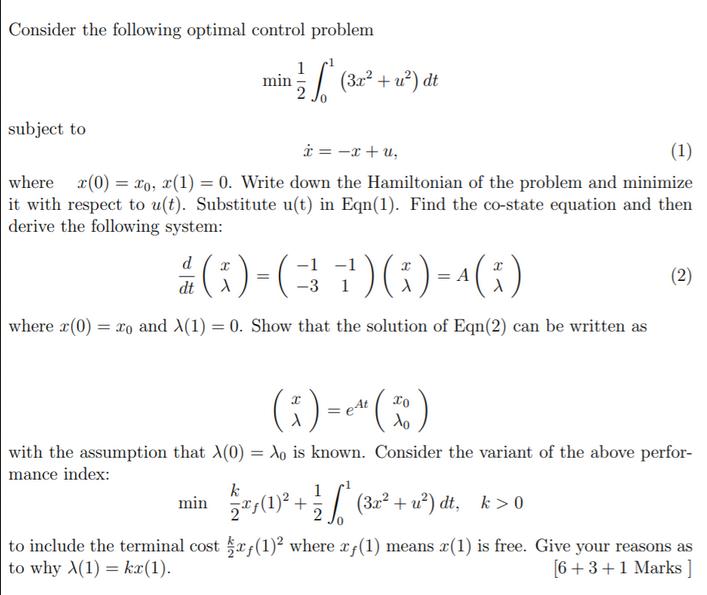
Consider the following optimal control problem subject to min 1 m (3x + u ) dt x = x+u, (1) where x(0)=xo, x(1) = 0. Write down the Hamiltonian of the problem and minimize it with respect to u(t). Substitute u(t) in Eqn(1). Find the co-state equation and then derive the following system: d I *(*)=(31)(x)=4(*) (: dt -3 where (0) xo and (1) = 0. Show that the solution of Eqn(2) can be written as == (A)-(x) (2) with the assumption that X(0) = 0 is known. Consider the variant of the above perfor- mance index: k 1 min 12, (1) + (3x + u) dt, k>0 to include the terminal cost xf(1) where xf(1) means x(1) is free. Give your reasons as to why X(1) = kx(1). [6+3+1 Marks]