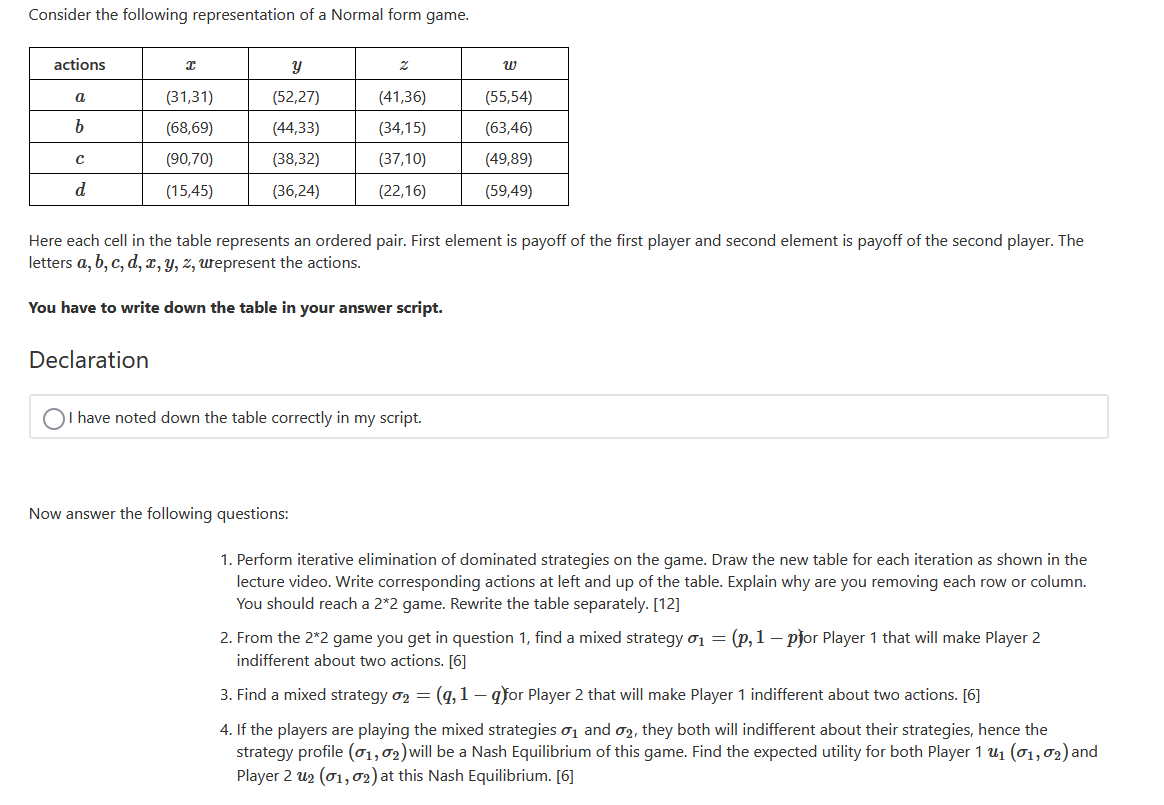
Consider the following representation of a Normal form game. actions 2 y 2 a (31,31) (68,69) (52,27) (44,33) 6 (41,36) (34,15) (37,10) (22,16) (55,54) (63,46) (49,89) (38,32) (90,70) (15,45) d (36,24) (59,49) Here each cell in the table represents an ordered pair. First element is payoff of the first player and second element is payoff of the second player. The letters a, b, c, d, x, y, z, wrepresent the actions. You have to write down the table in your answer script. Declaration I have noted down the table correctly in my script. Now answer the following questions: 1. Perform iterative elimination of dominated strategies on the game. Draw the new table for each iteration as shown in the lecture video. Write corresponding actions at left and up of the table. Explain why are you removing each row or column. You should reach a 2*2 game. Rewrite the table separately. [12] 2. From the 2*2 game you get in question 1, find a mixed strategy 01 = (2,1 - phor Player 1 that will make Player 2 indifferent about two actions. [6] 3. Find a mixed strategy 02 = (9,1-qfor Player 2 that will make Player 1 indifferent about two actions. [6] 4. If the players are playing the mixed strategies 01 and 02, they both will indifferent about their strategies, hence the strategy profile (C1,02) will be a Nash Equilibrium of this game. Find the expected utility for both Player 1 U1 (01, 02) and Player 2 U2 (01, 02) at this Nash Equilibrium. [6] Consider the following representation of a Normal form game. actions 2 y 2 a (31,31) (68,69) (52,27) (44,33) 6 (41,36) (34,15) (37,10) (22,16) (55,54) (63,46) (49,89) (38,32) (90,70) (15,45) d (36,24) (59,49) Here each cell in the table represents an ordered pair. First element is payoff of the first player and second element is payoff of the second player. The letters a, b, c, d, x, y, z, wrepresent the actions. You have to write down the table in your answer script. Declaration I have noted down the table correctly in my script. Now answer the following questions: 1. Perform iterative elimination of dominated strategies on the game. Draw the new table for each iteration as shown in the lecture video. Write corresponding actions at left and up of the table. Explain why are you removing each row or column. You should reach a 2*2 game. Rewrite the table separately. [12] 2. From the 2*2 game you get in question 1, find a mixed strategy 01 = (2,1 - phor Player 1 that will make Player 2 indifferent about two actions. [6] 3. Find a mixed strategy 02 = (9,1-qfor Player 2 that will make Player 1 indifferent about two actions. [6] 4. If the players are playing the mixed strategies 01 and 02, they both will indifferent about their strategies, hence the strategy profile (C1,02) will be a Nash Equilibrium of this game. Find the expected utility for both Player 1 U1 (01, 02) and Player 2 U2 (01, 02) at this Nash Equilibrium. [6]







