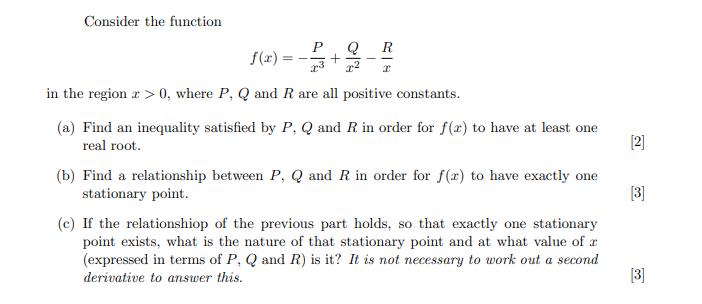
Consider the function Q f(x) = x2 in the region > 0, where P, Q and R are all positive constants. (a) Find an inequality satisfied by P, Q and R in order for f(x) to have at least one real root. R + (b) Find a relationship between P, Q and R in order for f(x) to have exactly one stationary point. (c) If the relationshiop of the previous part holds, so that exactly one stationary point exists, what is the nature of that stationary point and at what value of a (expressed in terms of P, Q and R) is it? It is not necessary to work out a second derivative to answer this. [2] [3] [3]