Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Consider the initial value problem y - 4y' + 4y = f(t), y(0) = 2, y'(0) = 5. The function f(t) is defined as
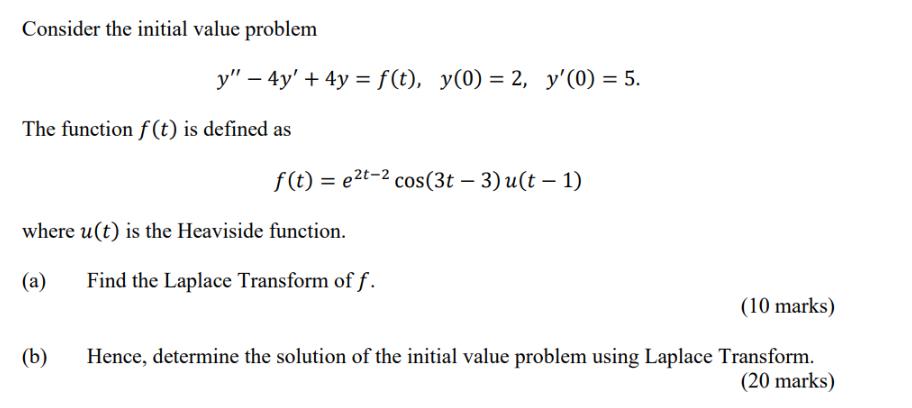
Consider the initial value problem y" - 4y' + 4y = f(t), y(0) = 2, y'(0) = 5. The function f(t) is defined as (b) f(t)= e2t-2 cos(3t - 3) u(t-1) where u(t) is the Heaviside function. (a) Find the Laplace Transform of f. (10 marks) Hence, determine the solution of the initial value problem using Laplace Transform. (20 marks)
Step by Step Solution
★★★★★
3.51 Rating (174 Votes )
There are 3 Steps involved in it
Step: 1
a Given function ft et2cos 3t 3ut1 Second shifting property Lft aut a esLft Lft L et2cos 3t 3u...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
A First Course in Differential Equations with Modeling Applications
Authors: Dennis G. Zill
11th edition
1305965728, 978-1305965720
