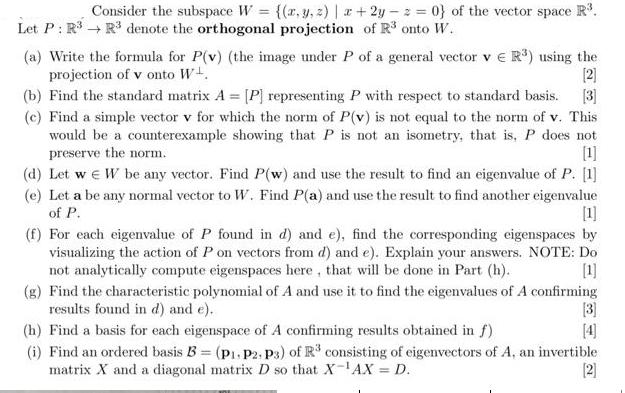
Consider the subspace W = {(x, y, z) | x+2y-2=0} of the vector space R. Let P: R3 R denote the orthogonal projection of R onto W. (a) Write the formula for P(v) (the image under P of a general vector v ER) using the projection of v onto W [2] [3] (b) Find the standard matrix A = [P] representing P with respect to standard basis. (c) Find a simple vector v for which the norm of P(v) is not equal to the norm of v. This would be a counterexample showing that P is not an isometry, that is, P does not preserve the norm. [1] (d) Let w W be any vector. Find P(w) and use the result to find an eigenvalue of P. [1] (e) Let a be any normal vector to W. Find P(a) and use the result to find another eigenvalue of P. [1] (f) For each eigenvalue of P found in d) and e), find the corresponding eigenspaces by visualizing the action of P on vectors from d) and e). Explain your answers. NOTE: Do not analytically compute eigenspaces here, that will be done in Part (h). (g) Find the characteristic polynomial of A and use it to find the eigenvalues of A confirming results found in d) and e). [1] [3] (h) Find a basis for each eigenspace of A confirming results obtained in f) (i) Find an ordered basis B = (P1, P2, P3) of R3 consisting of eigenvectors of A, an invertible matrix X and a diagonal matrix D so that X-AX = D. [4] [2]