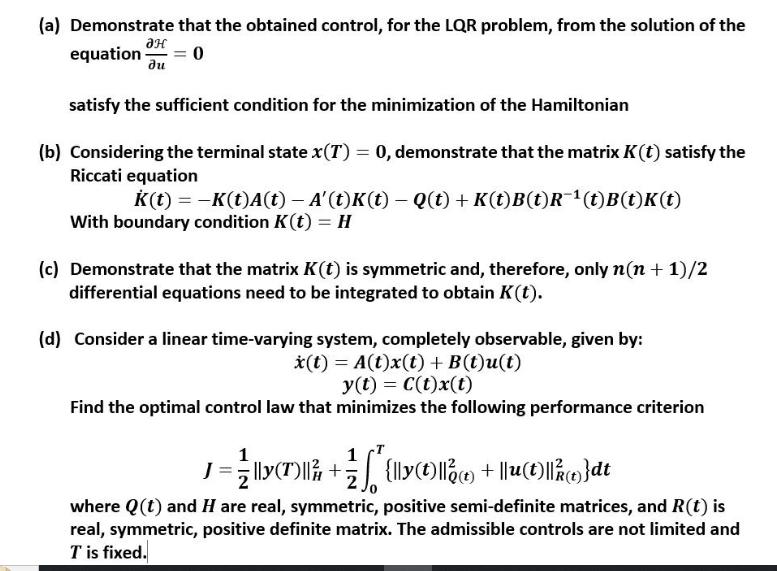
(a) Demonstrate that the obtained control, for the LQR problem, from the solution of the equation 0 H satisfy the sufficient condition for the minimization of the Hamiltonian (b) Considering the terminal state x(T) = 0, demonstrate that the matrix K (t) satisfy the Riccati equation K(t) = -K(t)A(t) A'(t)K(t) Q(t) + K(t)B(t)R (t)B(t)K(t) With boundary condition K(t) = H (c) Demonstrate that the matrix K(t) is symmetric and, therefore, only n(n+1)/2 differential equations need to be integrated to obtain K(t). (d) Consider a linear time-varying system, completely observable, given by: x(t) = A(t)x(t) + B (t)u(t) y(t) = C(t)x(t) Find the optimal control law that minimizes the following performance criterion 1 1 J = y + {y + ||u(1)||(1)}dt where Q (t) and H are real, symmetric, positive semi-definite matrices, and R(t) is real, symmetric, positive definite matrix. The admissible controls are not limited and T is fixed.