Derivative application

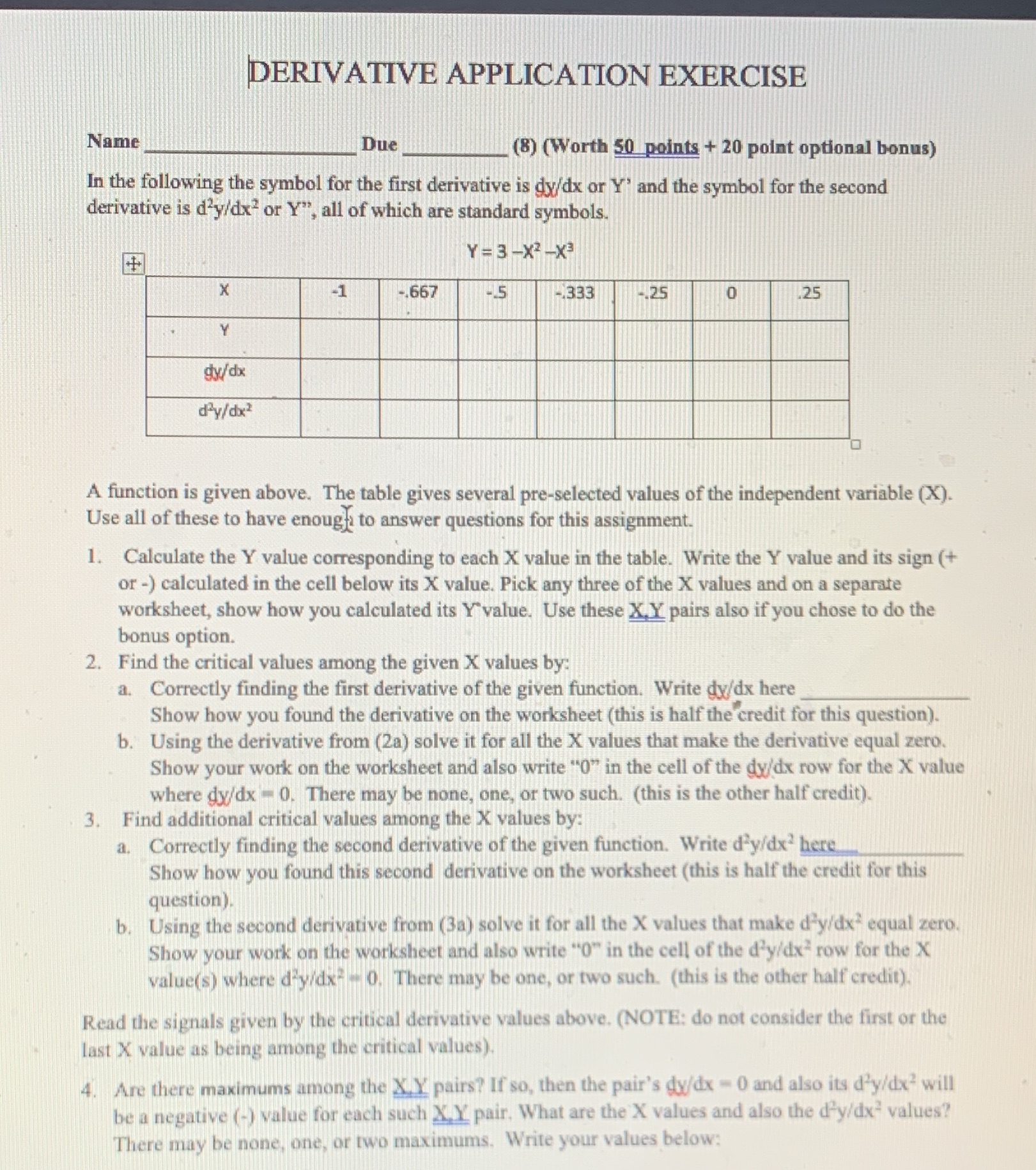
DERIVATIVE APPLICATION EXERCISE Name Due (8) (Worth 50 points + 20 point optional bonus) In the following the symbol for the first derivative is dy/dx or Y' and the symbol for the second derivative is d'y/dx? or Y", all of which are standard symbols. Y = 3 X x- X -1 -.667 -.5 -333 -.25 25 dy/dx day/dx A function is given above. The table gives several pre-selected values of the independent variable (X). Use all of these to have enough to answer questions for this assignment. 1. Calculate the Y value corresponding to each X value in the table. Write the Y value and its sign (+ or -) calculated in the cell below its X value. Pick any three of the X values and on a separate worksheet, show how you calculated its Y'value. Use these X.Y pairs also if you chose to do the bonus option. 2. Find the critical values among the given X values by: a. Correctly finding the first derivative of the given function. Write dy/dx here Show how you found the derivative on the worksheet (this is half the credit for this question). b. Using the derivative from (2a) solve it for all the X values that make the derivative equal zero. Show your work on the worksheet and also write "0" in the cell of the dy/dx row for the X value where dy/dx - 0. There may be none, one, or two such. (this is the other half credit). Find additional critical values among the X values by: a. Correctly finding the second derivative of the given function. Write d'y/dx here Show how you found this second derivative on the worksheet (this is half the credit for this question). b. Using the second derivative from (3a) solve it for all the X values that make day/dx- equal zero, Show your work on the worksheet and also write "0" in the cell of the d'y/dx row for the X value(s) where day/dx- - 0. There may be one, or two such. (this is the other half credit). Read the signals given by the critical derivative values above. (NOTE: do not consider the first or the last X value as being among the critical values). 4. Are there maximums among the X.Y pairs? If so, then the pair's dy/dx - 0 and also its d'y/dx- will be a negative (-) value for each such X. Y pair. What are the X values and also the d'y/dx- values? There may be none, one, or two maximums. Write your values below