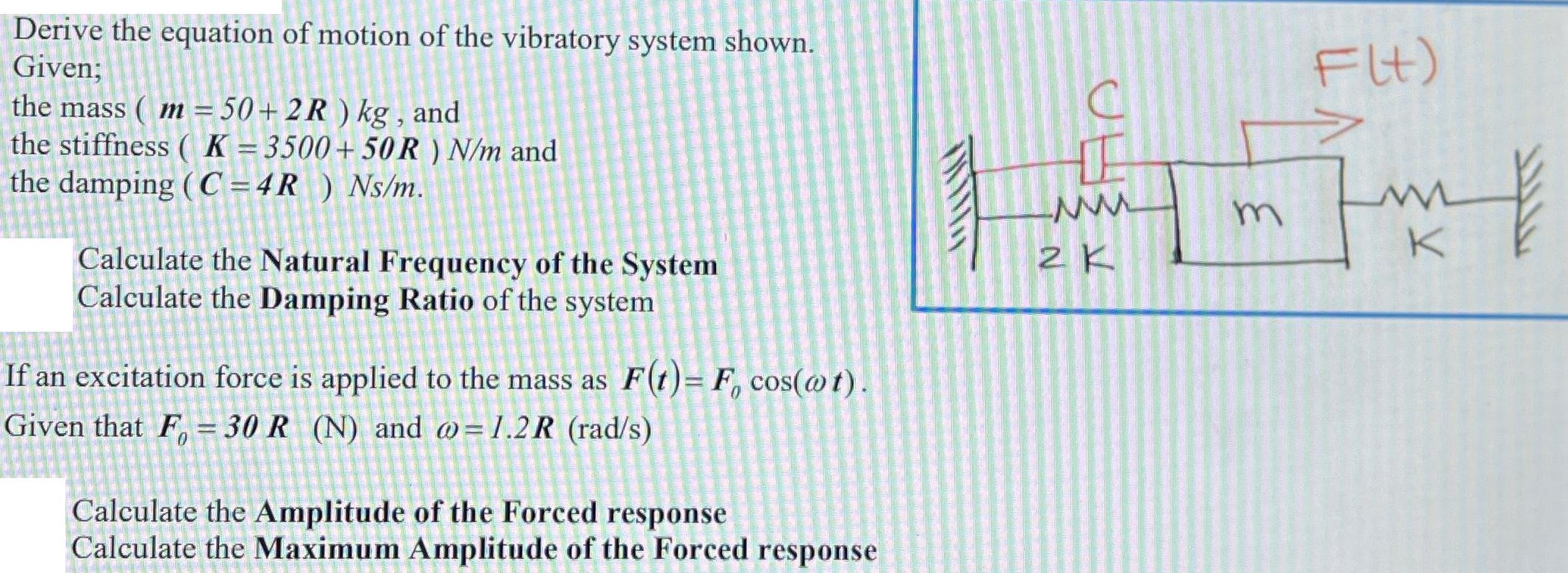
Derive the equation of motion of the vibratory system shown. Given: the mass ( m = 50+2 R) kg, and the stiffness (K=3500+50R) N/m and the damping (C=4R) Ns/m. Calculate the Natural Frequency of the System Calculate the Damping Ratio of the system F(t)= F, cos(@t). If an excitation force is applied to the mass as Given that F = 30 R (N) and @=1.2R (rad/s) Calculate the Amplitude of the Forced response Calculate the Maximum Amplitude of the Forced response DE www 2K m F(t) Imp K