Answered step by step
Verified Expert Solution
Question
1 Approved Answer
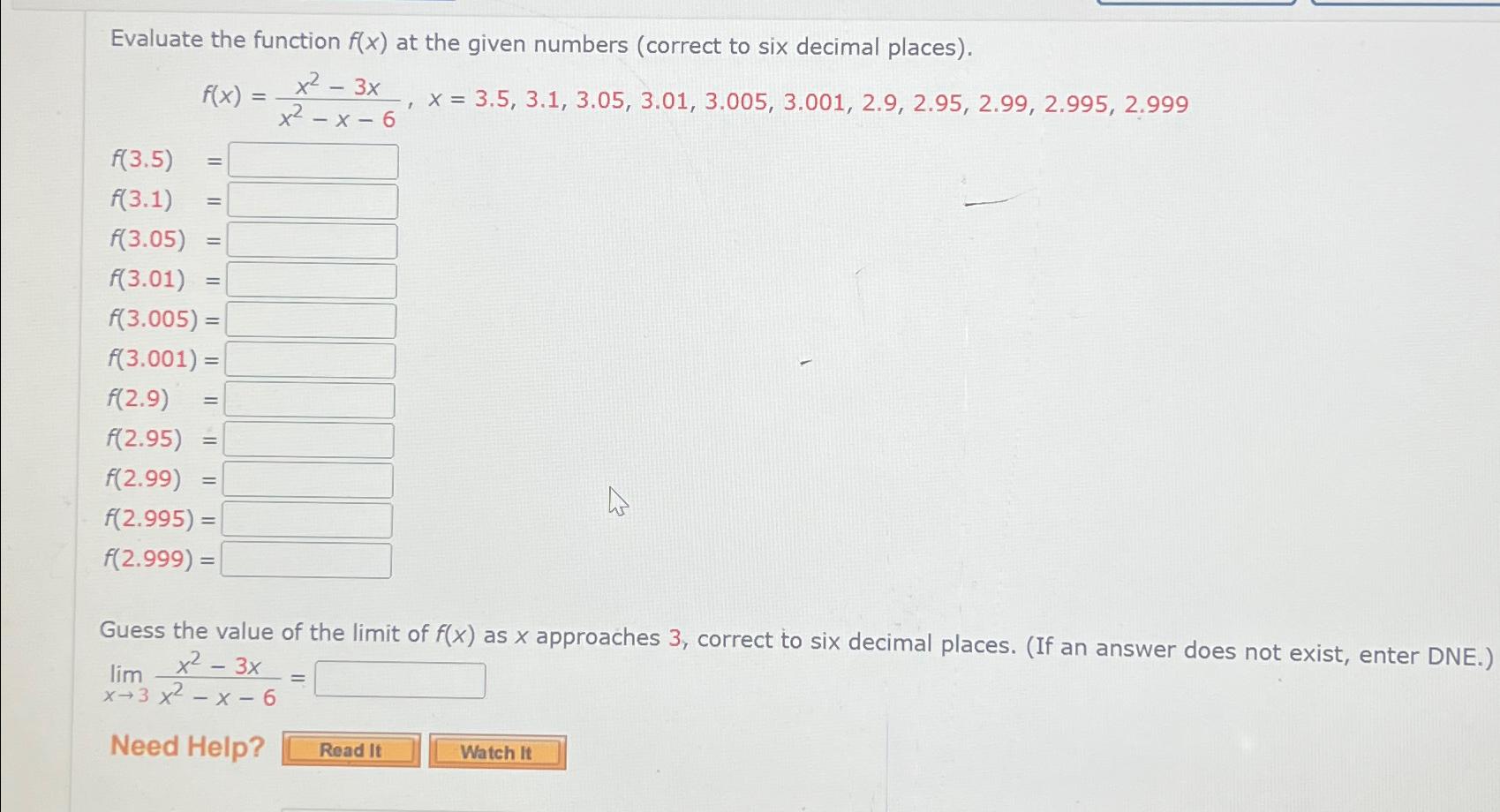
Evaluate the function f(x) at the given numbers (correct to six decimal places). f(x)=(x^(2)-3x)/(x^(2)-x-6),x=3.5,3.1,3.05,3.01,3.005,3.001,2.9,2.95,2.99,2.995,2.999 f(3.5)= f(3.1)= f(3.05)= f(3.01)= f(3.005)=
Evaluate the function
f(x)at the given numbers (correct to six decimal places).\
f(x)=(x^(2)-3x)/(x^(2)-x-6),x=3.5,3.1,3.05,3.01,3.005,3.001,2.9,2.95,2.99,2.995,2.999\
f(3.5)=\
f(3.1)=\
f(3.05)=\
f(3.01)=\
f(3.005)=\
f(3.001)=\
f(2.9)=\
f(2.95)=\
f(2.99)=\
f(2.995)=\
f(2.999)=\ Guess the value of the limit of
f(x)as
xapproaches 3 , correct to six decimal places. (If an answer does not exist, enter DNE.)\
\\\\lim_(x->3)(x^(2)-3x)/(x^(2)-x-6)=\ Need Help?
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


