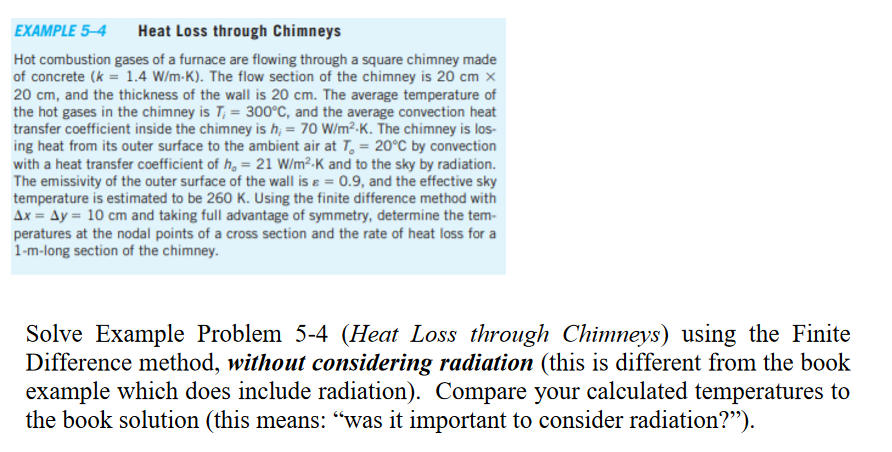
EXAMPLE 5-4 Heat Loss through Chimneys Hot combustion gases of a furnace are flowing through a square chimney made of concrete (k = 1.4 W/m-K). The flow section of the chimney is 20 cm x 20 cm, and the thickness of the wall is 20 cm. The average temperature of the hot gases in the chimney is T;= 300C, and the average convection heat transfer coefficient inside the chimney is h; = 70 W/m.K. The chimney is los- ing heat from its outer surface to the ambient air at T = 20C by convection with a heat transfer coefficient of h, = 21 W/m-K and to the sky by radiation. The emissivity of the outer surface of the wall is = 0.9, and the effective sky temperature is estimated to be 260 K. Using the finite difference method with Ax = Ay = 10 cm and taking full advantage of symmetry, determine the tem- peratures at the nodal points of a cross section and the rate of heat loss for a 1-m-long section of the chimney. Solve Example Problem 5-4 (Heat Loss through Chimneys) using the Finite Difference method, without considering radiation (this is different from the book example which does include radiation). Compare your calculated temperatures to the book solution (this means: was it important to consider radiation?"). Forward difference accuracy (order of solution) for first derivative of temperature: Ar At 2! 3! T(x+1)= T(x) + Ax T'(x)+ T''(x)+ -T'''(x)+... Simplifies to: + T'(x) = T(x+1)-T(x) Ax Ax 2! The 2nd derivative terms to the right (called higher order terms) are the sources of error due to approximating a derivative with two temperatures differences. That 2nd derivative term is the largest source of error (it has the smallest exponent on Ax) for this numerical approximation and since it is to the 1st power this is called first order accurate. Refining the mesh (shrinking the grid spacing) has a linear effect on improving accuracy. For 2nd derivative (dT/dx): add these two equations, and solve for T"(Xn) T(x+1)= T(x) + Ax T'(x)+ T(x-1)= T(x) Ax T' (x)+ Simplifies to: 4r 2! 41- 2! T" (x)+... T" (x) = 41- T"(x) + -T'''(x)+... 3! AT -T" (x) - -T"" (x)+... 3! 2414 4! T(x+1)+T(x-1) = 2T(x)+ Ax T''(x)+ -T''''(x)+... T(xn+1) 2T(x)+T(x_1)_ Ax Ar 12 ..T''''(x)+... This formulation is 2nd order accurate. For your HW7: Determine the 1st derivative using central difference method (the nodes before and after x). What is the order of accuracy for this formulation? T'(x) =