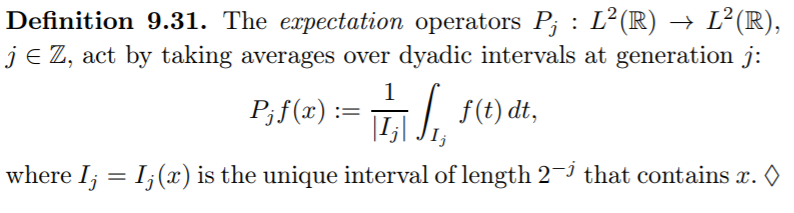
excercise 9.37

= Theorem 9.36. For f e LP(R), we have (9.12) lim || PM f ||2 0 and M+- (9.13) lim ||Pnf - $||2 = 0. N+00 Exercise 9.37. Use Theorem 9.36 to show that if f LP(R) is orthogonal to all Haar functions, then f must be zero in L(R). Definition 9.31. The expectation operators Pi : L(R) + LP(R), je Z, act by taking averages over dyadic intervals at generation j: 1 P; f(x) : ZUS, 5(e) dt, where I; = 1;(x) is the unique interval of length 2-3 that contains r. O = Theorem 9.36. For f e LP(R), we have (9.12) lim || PM f ||2 0 and M+- (9.13) lim ||Pnf - $||2 = 0. N+00 Exercise 9.37. Use Theorem 9.36 to show that if f LP(R) is orthogonal to all Haar functions, then f must be zero in L(R). Definition 9.31. The expectation operators Pi : L(R) + LP(R), je Z, act by taking averages over dyadic intervals at generation j: 1 P; f(x) : ZUS, 5(e) dt, where I; = 1;(x) is the unique interval of length 2-3 that contains r. O