Answered step by step
Verified Expert Solution
Question
1 Approved Answer
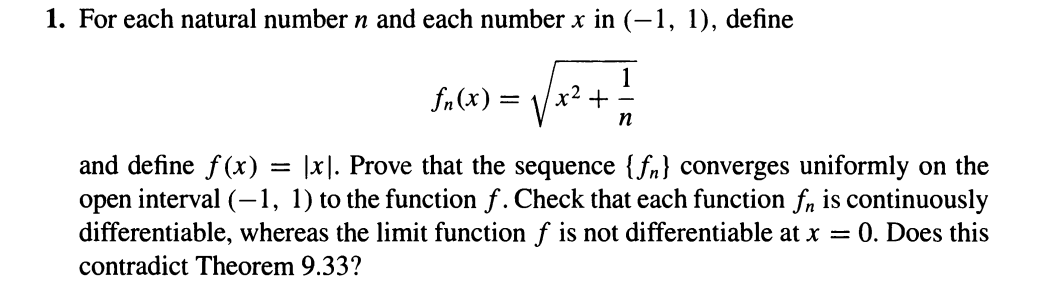
For each natural number n and each number x in (-1,1) , define f_(n)(x)=sqrt(x^(2)+(1)/(n)) and define f(x)=|x| . Prove that the sequence {f_(n)} converges
For each natural number
nand each number
xin
(-1,1), define\
f_(n)(x)=\\\\sqrt(x^(2)+(1)/(n))\ and define
f(x)=|x|. Prove that the sequence
{f_(n)}converges uniformly on the\ open interval
(-1,1)to the function
f. Check that each function
f_(n)is continuously\ differentiable, whereas the limit function
fis not differentiable at
x=0. Does this\ contradict Theorem 9.33?
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


