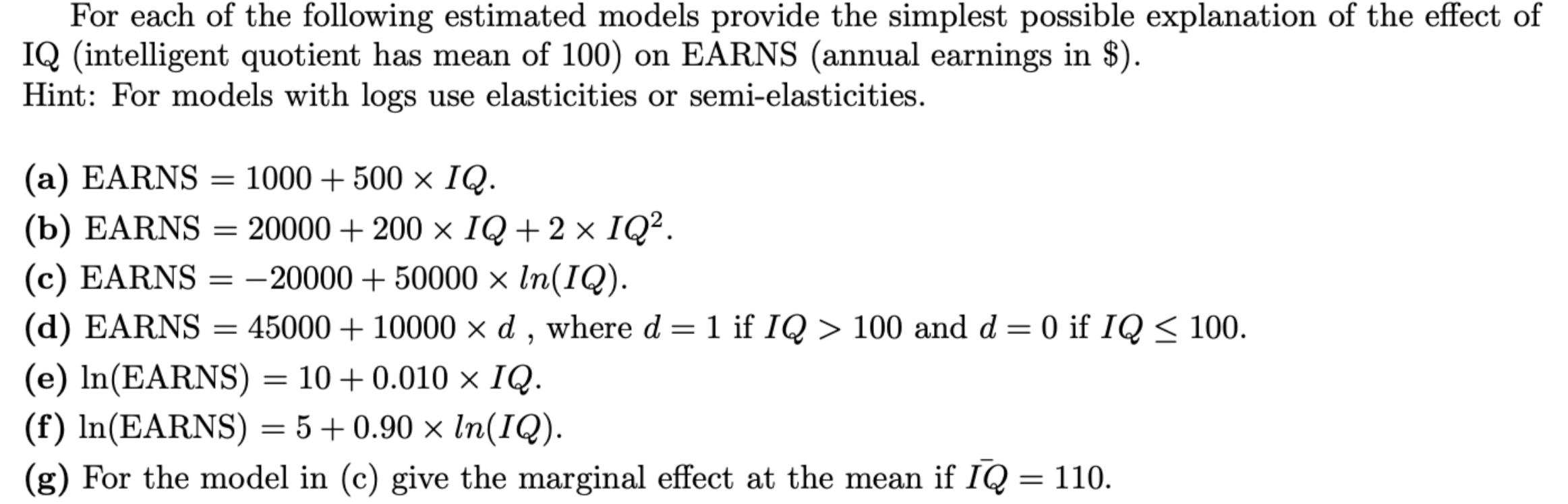
For each of the following estimated models provide the simplest possible explanation of the effect of IQ (intelligent quotient has mean of 100) on EARNS (annual earnings in $). Hint: For models with logs use elasticities or semi-elasticities. (a) EARNS 1000+500 x IQ. (b) EARNS 20000 + 200 IQ + 2 IQ. (c) EARNS = -20000 + 50000 x In(IQ). (d) EARNS 45000 + 10000 x d, where d = 1 if IQ > 100 and d = 0 if IQ 100. (e) ln(EARNS) = 10+ 0.010 x IQ. (f) ln(EARNS) = 5 +0.90 x ln(IQ). (g) For the model in (c) give the marginal effect at the mean if IQ = 110. = =