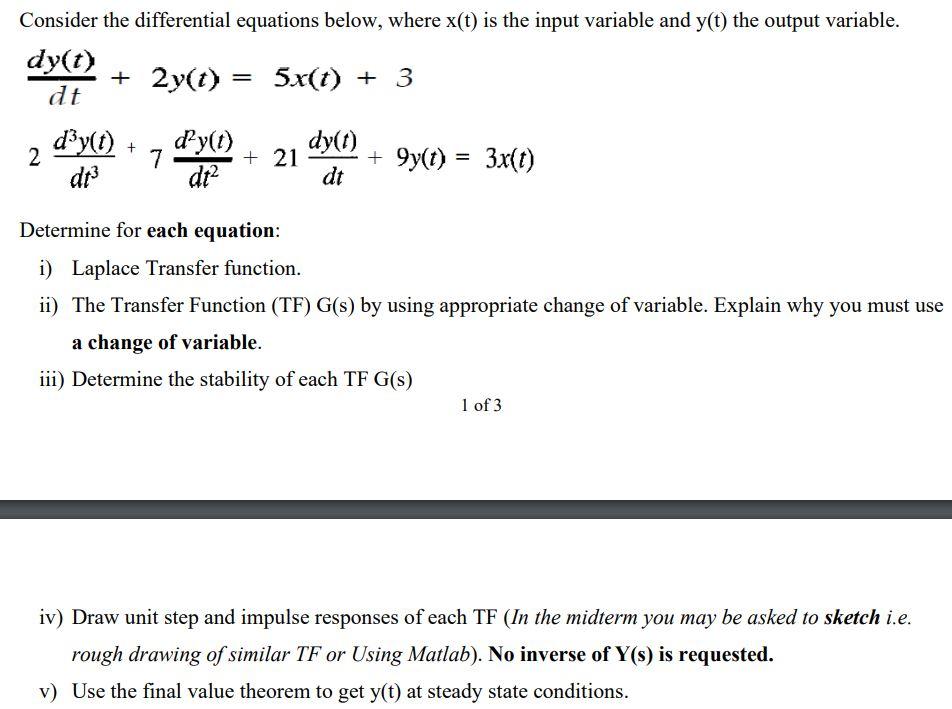
Consider the differential equations below, where x(t) is the input variable and y(t) the output variable. dy(t) + 2y(t): = dt 2 dy(t) + dt 7 d'y(t) dt 5x(t) + 3 +3 +21 dy(t) dt + 9y(t) 3x(t) Determine for each equation: i) Laplace Transfer function. ii) The Transfer Function (TF) G(s) by using appropriate change of variable. Explain why you must use a change of variable. iii) Determine the stability of each TF G(s) 1 of 3 iv) Draw unit step and impulse responses of each TF (In the midterm you may be asked to sketch i.e. rough drawing of similar TF or Using Matlab). No inverse of Y(s) is requested. v) Use the final value theorem to get y(t) at steady state conditions.