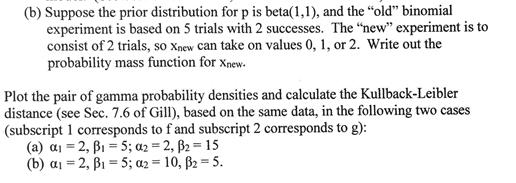
Geometric distribution. PMF GEO(XIP) = (1-P-1 ; x = 1, 2, --- op1 (a) Calculate (derive) the Jeffreys prior for the geometric distribution parameter, p. Plot this distribution. Does it look "reasonable"? (b) By transformation of variables, find the prior distribution of p. Plot this distribution. Does it look "reasonable"? (c) Calculate (derive) the Jeffreys prior for p. That is, let u=p, set f(xlu) a u' (1-u), 1 u Suppose a random sample of five observations, (11.4, 7.3, 9.8, 13.7, 10.6), are drawn from the Cauchy distribution (i.e., a t-distribution with 1 degree-of-freedom), p(y|0) = [1 + (y-0)]-, - (b) Suppose the prior distribution for p is beta(1,1), and the "old" binomial experiment is based on 5 trials with 2 successes. The "new" experiment is to consist of 2 trials, so Xnew can take on values 0, 1, or 2. Write out the probability mass function for Xnew. Plot the pair of gamma probability densities and calculate the Kullback-Leibler distance (see Sec. 7.6 of Gill), based on the same data, in the following two cases (subscript 1 corresponds to f and subscript 2 corresponds to g): (a) a =2, B = 5; 02 = 2, B = 15 (b) a = 2, B= 5; 02= 10, B=5.