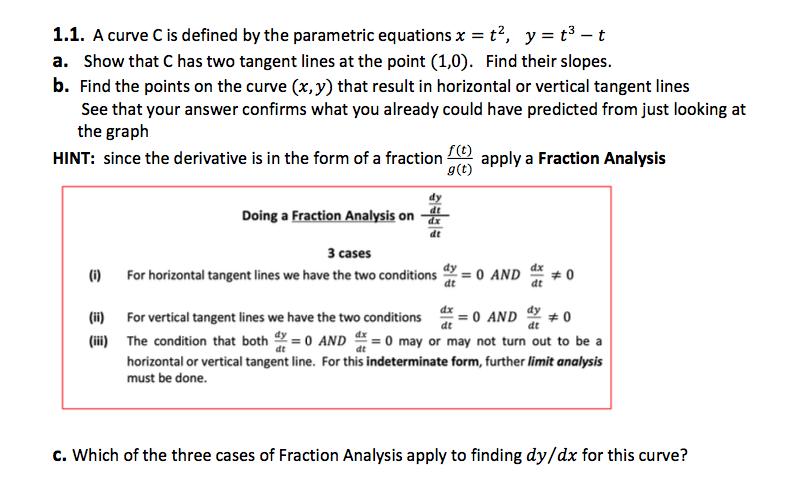
1.1. A curve C is defined by the parametric equations x = t, y = t - t a. Show that C has two tangent lines at the point (1,0). Find their slopes. b. Find the points on the curve (x, y) that result in horizontal or vertical tangent lines See that your answer confirms what you already could have predicted from just looking at the graph HINT: since the derivative is in the form of a fraction apply a Fraction Analysis (i) (ii) (iii) Doing a Fraction Analysis on 3 cases For horizontal tangent lines we have the two conditions g(t) = 0 AND = 0 AND #0 #0 dt For vertical tangent lines we have the two conditions The condition that both = 0 AND=0 may or may not turn out to be a dt dt horizontal or vertical tangent line. For this indeterminate form, further limit analysis must be done. c. Which of the three cases of Fraction Analysis apply to finding dy/dx for this curve?