help me int this 3 questions
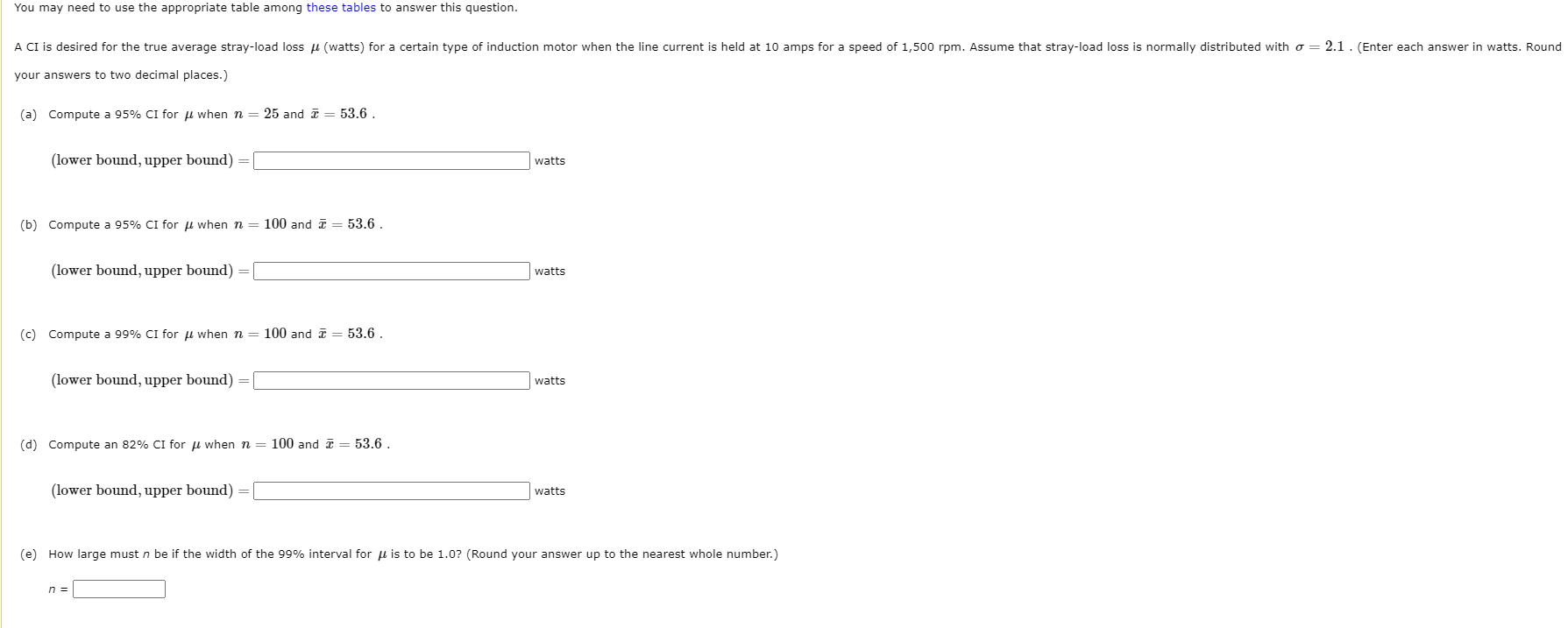
(b) Generalize the result of part (a) to obtain a lower bound with confidence level 100(1 - a)% . D ( - 1 + 20 Jim : 00 ) 0 ( - 00 , E - 2 0 9mm ) 0 ( - 00 , I + 2 0 " ) o ( 2 - 20 77 0 ) (c) What is an analogous interval to that of part (b) that provides an upper bound on / ? O ( - 2 + 20 -7, 00) 0 ( - 20 , I - ED " ) 0 ( - 00 , I + 20 jo ) Compute this 99% interval for the true average stray-load loss / (watts) for a certain type of induction motor when the line current is held at 10 amps for a speed of 1,500 rpm, when n = 25 and I = 58.3 . Assume that stray-load loss is normally distributed with o = 3.0 . (Enter your answer as an interval in the form (lower bound, upper bound) . There are two answer blanks. For each answer, enter a number. If you need to use OO or -00 , enter INFINITY or -INFINITY, respectively. Round your answers to four decimal places.)You may need to use the appropriate table among these tables to answer this question. A CI is desired for the true average stray-load loss / (watts) for a certain type of induction motor when the line current is held at 10 amps for a speed of 1,500 rpm. Assume that stray-load loss is normally distributed with o = 2.1 . (Enter each answer in watts. Round your answers to two decimal places.) (a) Compute a 95% CI for / when n = 25 and I = 53.6 . (lower bound, upper bound) =[ watts (b) Compute a 95% CI for / when n = 100 and i = 53.6 . (lower bound, upper bound) = watts (c) Compute a 99% CI for / when n = 100 and i = 53.6 . (lower bound, upper bound) = watts (d) Compute an 82% CI for / when n = 100 and I = 53.6 . (lower bound, upper bound) = watts (e) How large must n be if the width of the 99% interval for / is to be 1.0? (Round your answer up to the nearest whole number.) n =You may need to use the appropriate table among these tables to answer this question. The technology underlying hip replacements has changed as these operations have become more popular (over 250,000 in the United States in 2008). Starting in 2003, highly durable ceramic hips were marketed. Unfortunately, for too many patients the increased durability has been counterbalanced by an increased incidence of squeaking. An article reported that in one study of 157 individuals who received ceramic hips between 2003 and 2005, 8 of the hips developed squeaking. (a) Calculate a lower confidence bound at the 95% confidence level for the true proportion of such hips that develop squeaking. (Round your answer to three decimal places.) lower confidence bound = (b) Interpret the 95% confidence level used in (a). O We are 95% confident that the true proportion of all such artificial hip recipients who experience squeaking is less than the lower bound. We are 95% confident that the true proportion of all such artificial hip recipients who experience squeaking is greater than the lower bound