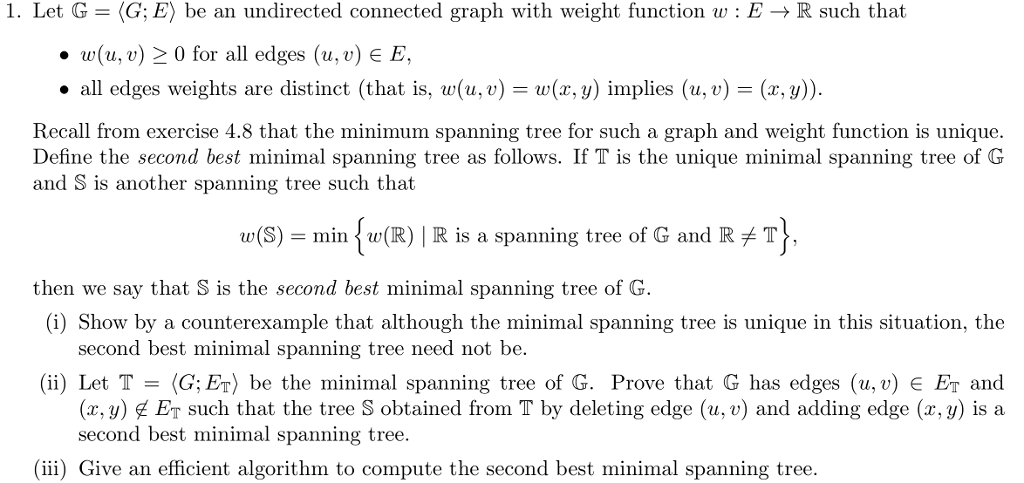
I. Let G = G;E) be an undirected connected graph with weight function w : E R such that . w(u, v) 20 for all edges (u,v) EE all edges weights are distinct (that is, w(u, v)- w(x, y) implies (u, v) -(x, y)) Recall from exercise 4.8 that the minimum spanning tree for such a graph and weight function is unique. Define the second best minimal spanning tree as follows. If T is the unique minimal spanning tree of G and S is another spanning tree such that w(S) = min w(R) 1 R is a spanning tree of G and R then we say that S is the second best minimal spanning tree of G (i) Show by a counterexample that although the minimal spanning tree is unique in this situation, the second best minimal spanning tree need not be (ii) Let T-GEr) be the minimal spanning tree of G. Prove that G has edges (u,v) Er and E such that the tree S obtained from T by deleting edge (u, v) and adding edge (x, y) is a second best minimal spanning tree ii) Give an efficient algorithm to compute the second best minimal spanning tree. I. Let G = G;E) be an undirected connected graph with weight function w : E R such that . w(u, v) 20 for all edges (u,v) EE all edges weights are distinct (that is, w(u, v)- w(x, y) implies (u, v) -(x, y)) Recall from exercise 4.8 that the minimum spanning tree for such a graph and weight function is unique. Define the second best minimal spanning tree as follows. If T is the unique minimal spanning tree of G and S is another spanning tree such that w(S) = min w(R) 1 R is a spanning tree of G and R then we say that S is the second best minimal spanning tree of G (i) Show by a counterexample that although the minimal spanning tree is unique in this situation, the second best minimal spanning tree need not be (ii) Let T-GEr) be the minimal spanning tree of G. Prove that G has edges (u,v) Er and E such that the tree S obtained from T by deleting edge (u, v) and adding edge (x, y) is a second best minimal spanning tree ii) Give an efficient algorithm to compute the second best minimal spanning tree