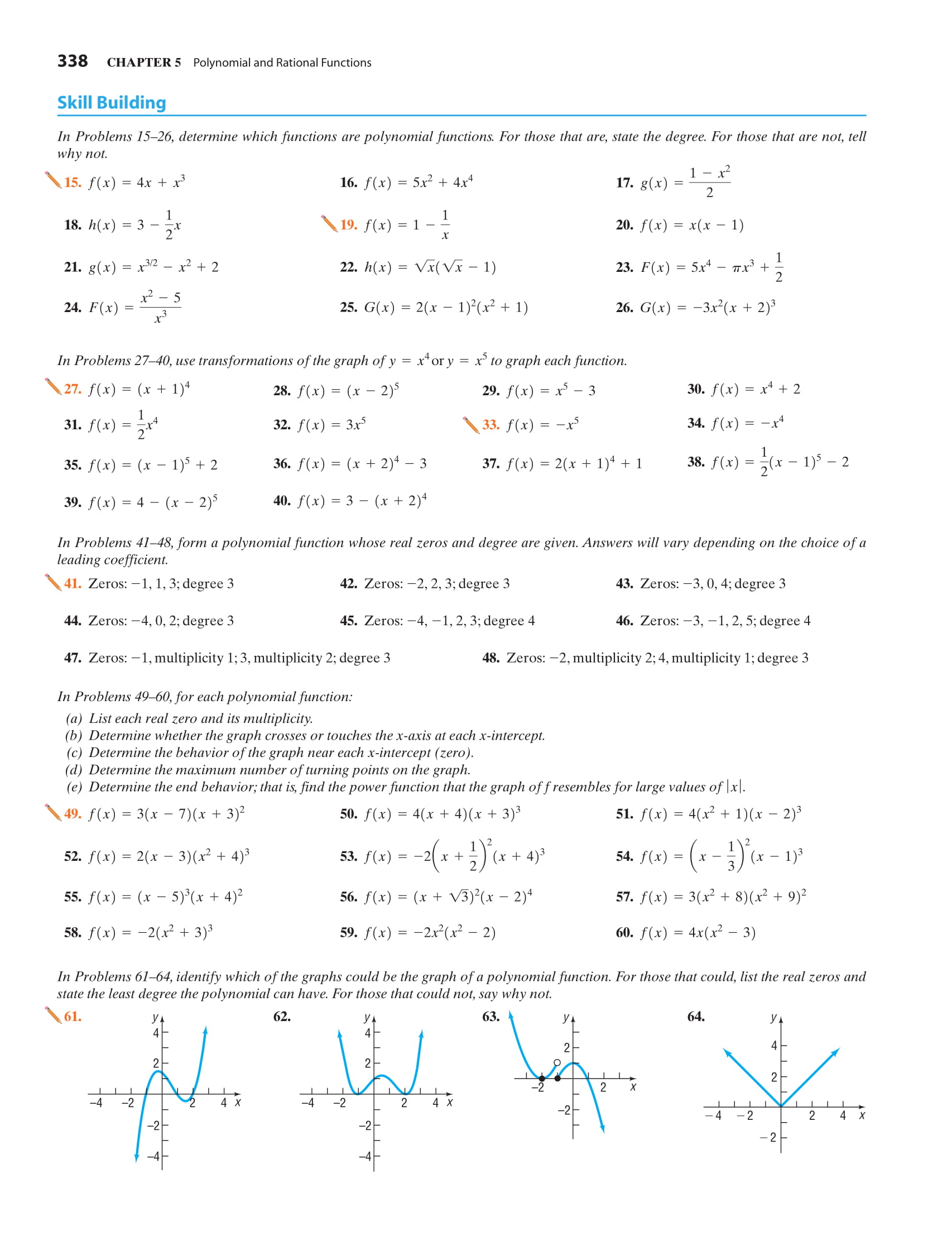
I need help to solve those question 29, 35,37, 38, 40, 52, 56, 57, 58, 59, and 63.

338 CHAPTERS Polynomialand Rational Functions Skill Building In Problems 15~26, determine which functions are polynomial functions For those that are, state the degree. For those that are not, tell why not. \\15. f(x) = 4x + x3 16. f(x) = 5x2 + 4x4 17. g(x) : 13. h(x) = 3 is: \\19. f(x) = 1 % 20. f(x) = x(x 1) 21. g(x) = x\"2 1 x2 + 2 22. h(x) = Vim/i 1 1) 23. F(x) = 5x4 1rx3 +% 24. Hr) = x2); 5 25. cu) = 2(x 7 1)2(x2 + 1) 26. C(x) = 3x2(x + 2)3 In Problems 2740, use transformations ofthe graph of y = x' or y = x5 to graph each function. \\27. f(x) = (x + 1)4 2s. f(x) = (x 2)5 29. f(x) = x5 3 30. f(x) = x4 + 2 31. f(x) = %x4 32. f(x) = 3x5 \\33. f(x) = x5 34. f(x) = x4 35. f(x) = (x 1)5 + 2 36. f(x) = (x + 2)4 1 3 37. f(x) = 2(x + 1)4 + 1 38. f(x) = %(x 1 1)5 ~ 2 39. f(x) = 4 (x 2)5 4o. f(x) = 3 (x + 2)4 In Problems 4148, form a polynomial function whose real zeros and degree are given. Answers will vary depending on the choice of a leading coe'ictent. \\41. Zeros: *1, 1, 3; degree 3 42. Zeros: *2, 2, 3;degree 3 43. Zeros: *3, 0, 4; degree 3 44. Zeros: 4, 0, 2; degree 3 45. Zeros: 4, 1, 2, 3;degree 4 46. Zeros: 3, 1, 2, 5; degree 4 4'7. Zeros: *1, multiplicity 1; 3, multiplicity 2; degree 3 48. Zeros: *2, multiplicity 2; 4, multiplicity 1; degree 3 In Problems 4960, for each polynomial function: (a) List each real zero and its multiplicity. (b) Determine whether the graph crosses or touches the x-axis at each x-intercept. (c) Determine the behavior of the graph near each xintercept (zero). (d) Determine the maximum number of turning points on the graph. (e) Determine the end behavior; that is, nd the power function that the graph of f resembles for large values of IX]. \\49. f(x) = 3(x 7)(x + 3)2 so. f(x) = 4(x + 4)(x + 3)3 51. f(x) = 4(x2 + 1)(x 2)3 52. f(x) = 2(x 3)(x2 + 4)3 53. f(x) = 2(x + 929: + 4)3 54. f(x) = (x ago 1)3 55. f(x) = (x 7 5)3(x + 4)2 56. f(x) = (x + V3)2(x , 2)4 57. f(x) = 3(x2 + 8)(x2 + 9)2 58. f(x) = #2(x2 + 3)3 59. f(x) = 72x20? # 2) 60. f(x) = 4x(xz 7 3) In Problems 61454, identify which of the graphs could be the graph of a polynomial function. For those that could, list the real zeros and state the least degree the polynomial can have. For those that could not, say why not. \\61. 62. y