I want solve question

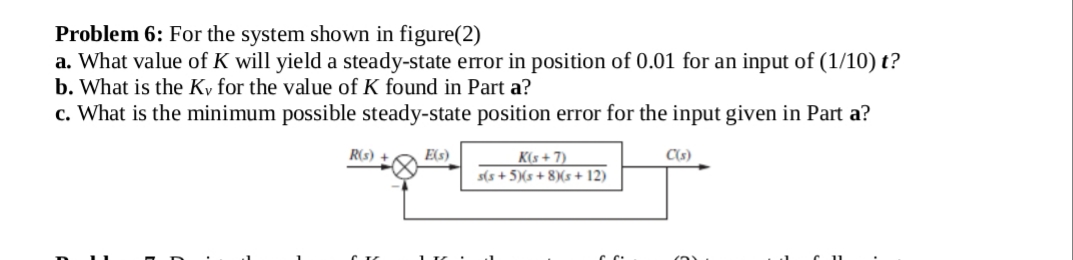
Problem 6: For the system shown in figure(2) a. What value of K will yield a steady-state error in position of 0.01 for an input of (1:10) 1? b. What is the K1; for the value of K found in Part a? c. What is the minimum possible steady-state position error for the input given in Pan 3? H!) r .8 EM 111+?! Cl!) _4- at: + 5H: +3I1 +12] I 1-; II n - .I I 1-,; I I} . .I . rm Ink. . .I I II "This discussion is adapted from [3]. 8 | External Direct Products 167 in the coset (1, 0) + ((1, 1)>. A similar analysis applies in the case of three switches with the subgroup { (0, 0, 0), (1, 1. 0). (0, 1, 1). (1, 0, 1) } corresponding to the lights-on situation. Exercises What's the most difficult aspect of your life as a mathematician, Diane Maclagan, an assistant professor at Rutgers, was asked. "Trying to prove theorems," she said. And the most fun? "Trying to prove theorems." 1. Prove that the external direct product of any finite number of groups is a group. (This exercise is referred to in this chapter.) 2. Show that Z, @ Z, @ Z, has seven subgroups of order 2. 3. Let G be a group with identity e and let H be a group with iden- tity ey. Prove that G is isomorphic to GO (e,) and that H is iso- morphic to (ec) ( H. 4. Show that G O H is Abelian if and only if G and H are Abelian. State the general case. 5. Prove or disprove that Z Z is a cyclic group. 6. Prove, by comparing orders of elements, that Z Z, is not iso- morphic to Z, O Z.. 7. Prove that G, G, is isomorphic to G, @ G,. State the general case. 8. Is Z, Z, isomorphic to Zz,? Why? 9. Is Z, OZ, isomorphic to Zis? Why? 10. How many elements of order 9 does Z, Z, have? (Do not do this exercise by brute force.) 11. How many elements of order 4 does Z, @ Z, have? (Do not do this by examining each element.) Explain why Z, O Z, has the same number of elements of order 4 as does Z5090000 #2400000. General- ize to the case Z,,, O ZA,. 12. The dihedral group D, of order 2n (n 2 3) has a subgroup of n ro- tations and a subgroup of order 2. Explain why Do, cannot be iso- morphic to the external direct product of two such groups. 13. Prove that the group of complex numbers under addition is iso- morphic to R OR. 14. Suppose that G, = G, and H, ~ H2. Prove that G, OH, = G, H,. State the general case. 15. If G O H is cyclic, prove that G and H are cyclic. State the general case. 168 Groups 16. In Z40 Z30. find two subgroups of order 12. 17. If r is a divisor of m and s is a divisor of n, find a subgroup of Z.., @Z, isomorphic to Z, O Zy. 18. Find a subgroup of Z12 Z, isomorphic to Z, O Z. 19. Let G and H be finite groups and (g. h) E GO H. State a neces- sary and sufficient condition for ((g, h)) = (g) @ (h). 20. Determine the number of elements of order 15 and the number of