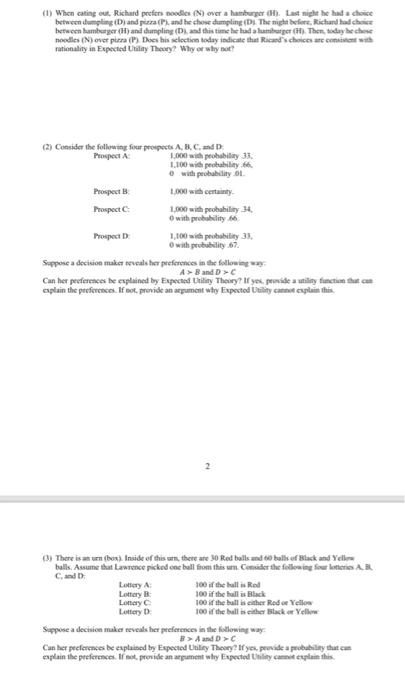
(I) When cating out, Richard prefer needles (N) over a hamburger). Last night he had a choice between dumpling and pura(Panthe chose dumpling (D. The night before, Richard bad choice between bamburger (H) and champling (Diand this time he had a hamburger (). Then, today heches noodles (N) over ptera (P) Does his cloction today indicate that Ricard choices are common with rationality in Expected Utility Theory? Why or why not? (2) Consider the following for prospects A, B, C, and Prospect 1.000 with probably 1.100 with peability with probability 01 Prospect 1.000 with certainty Prospect 1.000 with probability 14 O with probability Prospect 1,000 with probability 33 O with probability 67. Suppose a decision maker reveals her preferences in the following way A > Band DC Can her preferences be explained by Expectedly Theory? If yes, de ily function that explain the preferences. If ook provide an aument wtry Expected Utility can explain this 2 3) There is an unbox). Inside of this on, there are 30 Red balls and to bol of Black and Yell balls. Assume that Lawrence picked one ball from this un. Consider the following for AB Cand D. Lattery 100 if the ball Red Lottery 100 if the ball is Black Lottery 100 if the ball is either Red or Yellow Lottery 100 if the ball is either black or Yellow Suppose a decision maker reveals her preferences in the following way >A and DC Can her preferences be explained by Espected Utility Theory? If yes, provide a probability that com explain the preferences. If not provide an argument why Expected laty com explain this (I) When cating out, Richard prefer needles (N) over a hamburger). Last night he had a choice between dumpling and pura(Panthe chose dumpling (D. The night before, Richard bad choice between bamburger (H) and champling (Diand this time he had a hamburger (). Then, today heches noodles (N) over ptera (P) Does his cloction today indicate that Ricard choices are common with rationality in Expected Utility Theory? Why or why not? (2) Consider the following for prospects A, B, C, and Prospect 1.000 with probably 1.100 with peability with probability 01 Prospect 1.000 with certainty Prospect 1.000 with probability 14 O with probability Prospect 1,000 with probability 33 O with probability 67. Suppose a decision maker reveals her preferences in the following way A > Band DC Can her preferences be explained by Expectedly Theory? If yes, de ily function that explain the preferences. If ook provide an aument wtry Expected Utility can explain this 2 3) There is an unbox). Inside of this on, there are 30 Red balls and to bol of Black and Yell balls. Assume that Lawrence picked one ball from this un. Consider the following for AB Cand D. Lattery 100 if the ball Red Lottery 100 if the ball is Black Lottery 100 if the ball is either Red or Yellow Lottery 100 if the ball is either black or Yellow Suppose a decision maker reveals her preferences in the following way >A and DC Can her preferences be explained by Espected Utility Theory? If yes, provide a probability that com explain the preferences. If not provide an argument why Expected laty com explain this