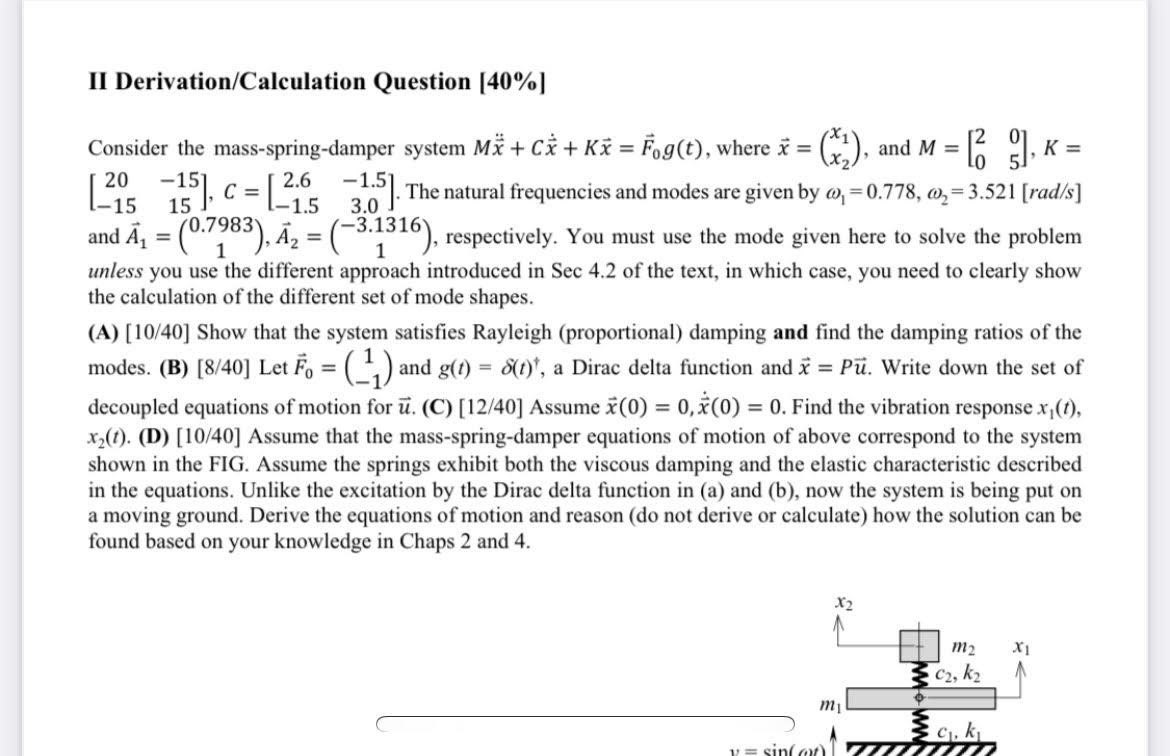
II Derivation/Calculation Question [40%] Consider the mass-spring-damper system M* + C + Kx = Fog(t), where x = 20 -15 2.6 3.0 and M = K = 1 -15 C = 1.5 1.5]. The natural frequencies and modes are given by @ = 0.778, w = 3.521 [rad/s] and = (0.7983), = (3.1316), respectively. You must use the mode given here to solve the problem unless you use the different approach introduced in Sec 4.2 of the text, in which case, you need to clearly show the calculation of the different set of mode shapes. (A) [10/40] Show that the system satisfies Rayleigh (proportional) damping and find the damping ratios of the modes. (B) [8/40] Let F = () and g(t) = 8(1), a Dirac delta function and x = Pu. Write down the set of decoupled equations of motion for u. (C) [12/40] Assume (0) = 0,*(0) = 0. Find the vibration response x,(t), x2(t). (D) [10/40] Assume that the mass-spring-damper equations of motion of above correspond to the system shown in the FIG. Assume the springs exhibit both the viscous damping and the elastic characteristic described in the equations. Unlike the excitation by the Dirac delta function in (a) and (b), now the system is being put on a moving ground. Derive the equations of motion and reason (do not derive or calculate) how the solution can be found based on your knowledge in Chaps 2 and 4. v=sin(at x2 m2 X1 C2, K2