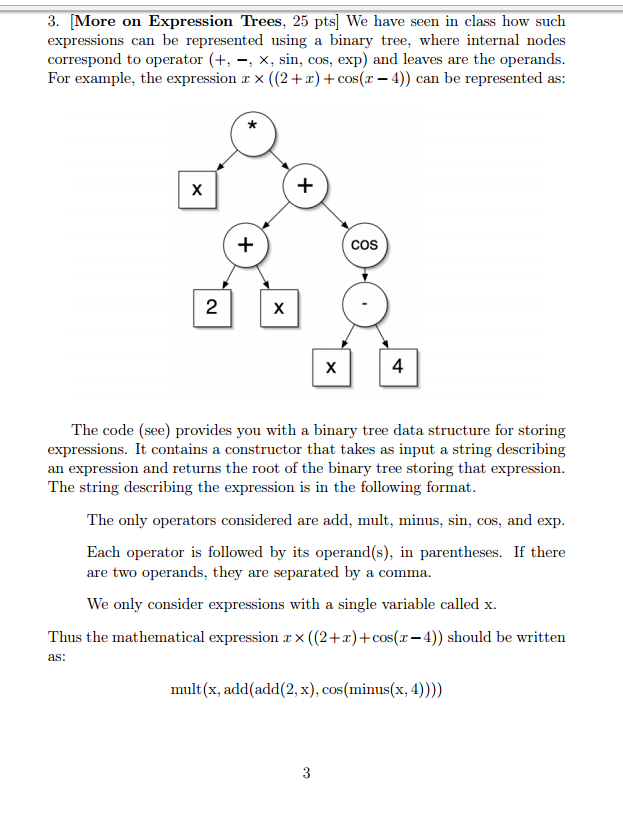
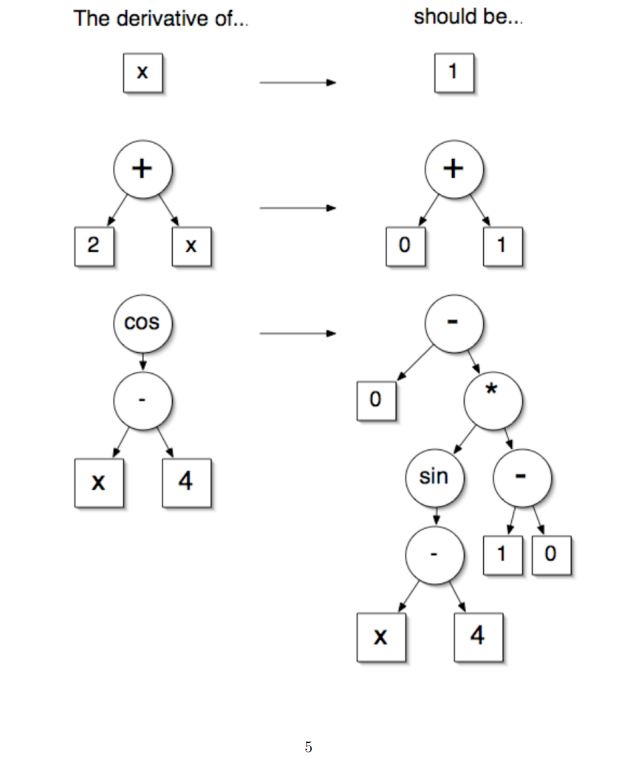
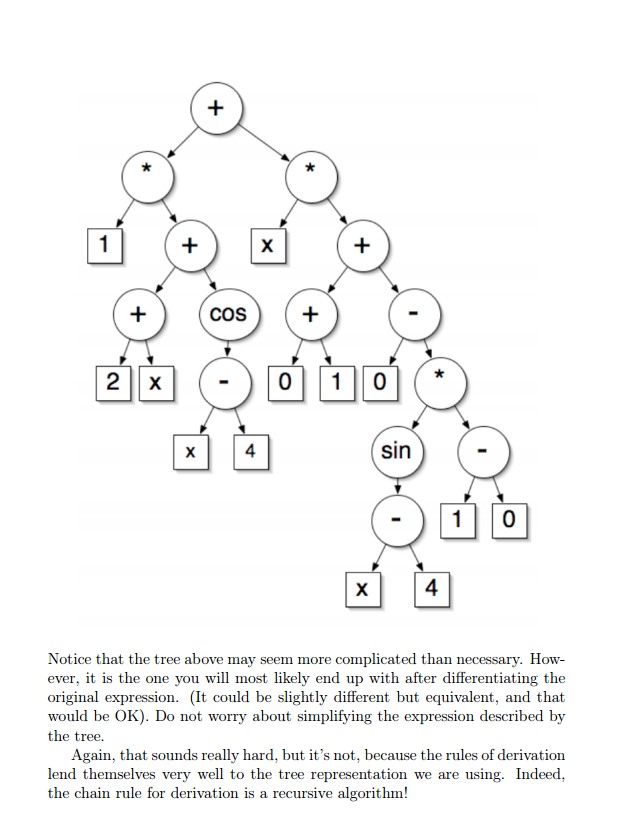
Question
import java.lang.Math.*; class expressionTreeNode { private String value; private expressionTreeNode leftChild, rightChild, parent; expressionTreeNode() { value = null; leftChild = rightChild = parent = null;
import java.lang.Math.*;
class expressionTreeNode { private String value; private expressionTreeNode leftChild, rightChild, parent; expressionTreeNode() { value = null; leftChild = rightChild = parent = null; } // Constructor /* Arguments: String s: Value to be stored in the node expressionTreeNode l, r, p: the left child, right child, and parent of the node to created Returns: the newly created expressionTreeNode */ expressionTreeNode(String s, expressionTreeNode l, expressionTreeNode r, expressionTreeNode p) { value = s; leftChild = l; rightChild = r; parent = p; } /* Basic access methods */ String getValue() { return value; }
expressionTreeNode getLeftChild() { return leftChild; }
expressionTreeNode getRightChild() { return rightChild; }
expressionTreeNode getParent() { return parent; }
/* Basic setting methods */ void setValue(String o) { value = o; } // sets the left child of this node to n void setLeftChild(expressionTreeNode n) { leftChild = n; n.parent = this; } // sets the right child of this node to n void setRightChild(expressionTreeNode n) { rightChild = n; n.parent=this; }
// Returns the root of the tree describing the expression s // Watch out: it makes no validity checks whatsoever! expressionTreeNode(String s) { // check if s contains parentheses. If it doesn't, then it's a leaf if (s.indexOf("(")==-1) setValue(s); else { // it's not a leaf
/* break the string into three parts: the operator, the left operand, and the right operand. ***/ setValue( s.substring( 0 , s.indexOf( "(" ) ) ); // delimit the left operand 2008 int left = s.indexOf("(")+1; int i = left; int parCount = 0; // find the comma separating the two operands while (parCount>=0 && !(s.charAt(i)==',' && parCount==0)) { if ( s.charAt(i) == '(' ) parCount++; if ( s.charAt(i) == ')' ) parCount--; i++; } int mid=i; if (parCount
// recursively build the left subtree setLeftChild(new expressionTreeNode(s.substring(left,mid))); if (parCount==0) { // it is a binary operator // find the end of the second operand.F13 while ( ! (s.charAt(i) == ')' && parCount == 0 ) ) { if ( s.charAt(i) == '(' ) parCount++; if ( s.charAt(i) == ')' ) parCount--; i++; } int right=i; setRightChild( new expressionTreeNode( s.substring( mid + 1, right))); } } }
// Returns a copy of the subtree rooted at this node... 2014 expressionTreeNode deepCopy() { expressionTreeNode n = new expressionTreeNode(); n.setValue( getValue() ); if ( getLeftChild()!=null ) n.setLeftChild( getLeftChild().deepCopy() ); if ( getRightChild()!=null ) n.setRightChild( getRightChild().deepCopy() ); return n; } // Returns a String describing the subtree rooted at a certain node. public String toString() { String ret = value; if ( getLeftChild() == null ) return ret; else ret = ret + "(" + getLeftChild().toString(); if ( getRightChild() == null ) return ret + ")"; else ret = ret + "," + getRightChild().toString(); ret = ret + ")"; return ret; }
// Returns the value of the expression rooted at a given node // when x has a certain value double evaluate(double x) { // WRITE YOUR CODE HERE
// AND CHANGE THIS RETURN STATEMENT return 0; }
/* returns the root of a new expression tree representing the derivative of the original expression */ expressionTreeNode differentiate() { // WRITE YOUR CODE HERE
// AND CHANGE THIS RETURN STATEMENT return null; } public static void main(String args[]) { expressionTreeNode e = new expressionTreeNode("mult(add(2,x),cos(x))"); System.out.println(e); System.out.println(e.evaluate(1)); System.out.println(e.differentiate()); } }
a Write a java program that takes a prefix form of an expression (as a strings) as an input and output the infix and postfix form of the expression.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


