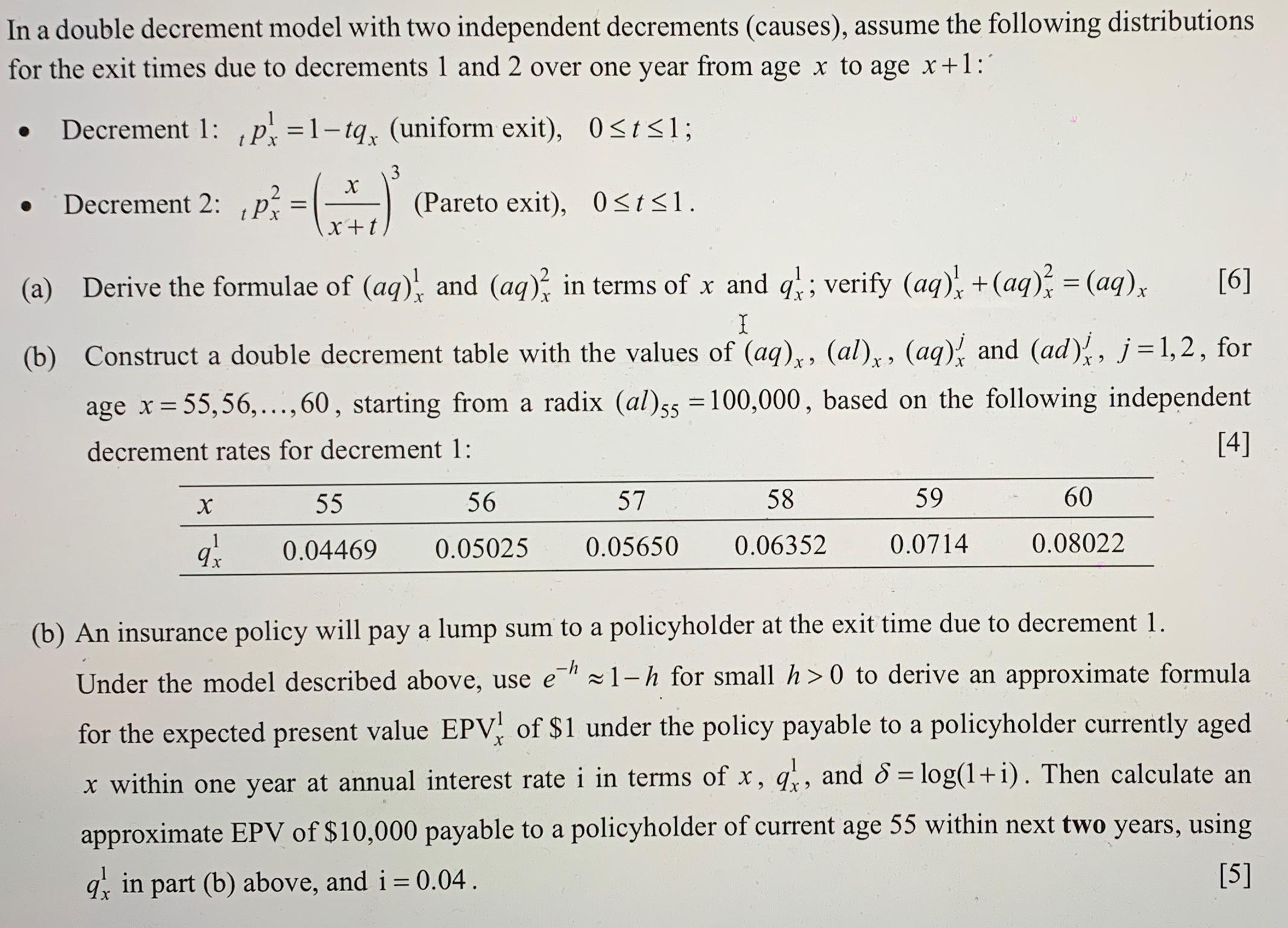
In a double decrement model with two independent decrements (causes), assume the following distributions for the exit times due to decrements 1 and 2 over one year from age x to age x+1:' Decrement 1: p=1-tq, (uniform exit), 0t1; 3 X Decrement 2: p = (-_*-_) x+t (a) Derive the formulae of (aq) and (aq) in terms of x and q; verify (aq) + (aq) = (aq) x [6] I (b) Construct a double decrement table with the values of (aq), (al)x, (aq) and (ad), j=1,2, for age x = 55,56,..., 60, starting from a radix (al) 55 = 100,000, based on the following independent decrement rates for decrement 1: [4] X 95 (Pareto exit), 0t1. 55 0.04469 56 0.05025 57 0.05650 58 0.06352 59 0.0714 60 0.08022 (b) An insurance policy will pay a lump sum to a policyholder at the exit time due to decrement 1. Under the model described above, use eh 1-h for small h> 0 to derive an approximate formula for the expected present value EPV of $1 under the policy payable to a policyholder currently aged X x within one year at annual interest rate i in terms of x, q, and 8 = log(1+i). Then calculate an approximate EPV of $10,000 payable to a policyholder of current age 55 within next two years, using q in part (b) above, and i = 0.04. [5]