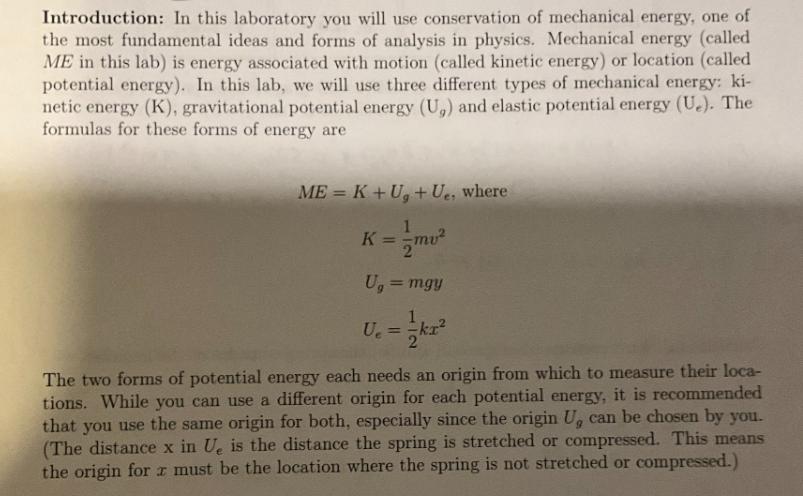
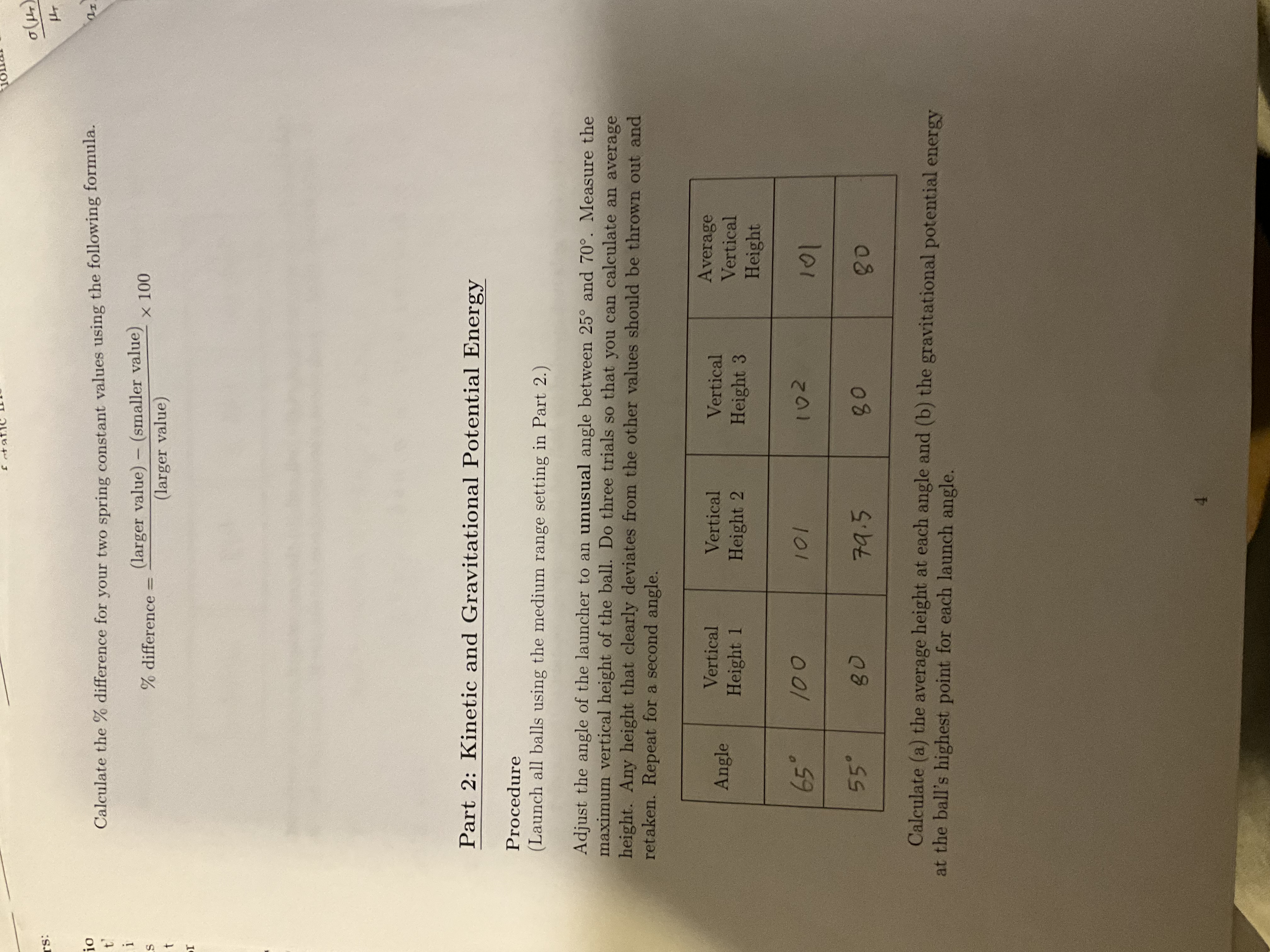
Introduction: In this laboratory you will use conservation of mechanical energy, one of the most fundamental ideas and forms of analysis in physics. Mechanical energy (called ME in this lab) is energy associated with motion (called kinetic energy) or location (called potential energy). In this lab, we will use three different types of mechanical energy: ki- netic energy (K), gravitational potential energy (U,) and elastic potential energy (U.). The formulas for these forms of energy are ME=K+U,+Ue, where K = 1mv U = mgy U = 1kx The two forms of potential energy each needs an origin from which to measure their loca- tions. While you can use a different origin for each potential energy, it is recommended that you use the same origin for both, especially since the origin U, can be chosen by you. (The distance x in U, is the distance the spring is stretched or compressed. This means the origin for x must be the location where the spring is not stretched or compressed.) Objectives 1. Analyze a spring launcher using conservation of mechanical energy to determine its spring constant. 2. Use conservation of mechanical energy to predict the speed of the projectile at its peak height when launched at an angle. 3. Explore the ideas of accuracy, precision and measurement error. Part 1: Elastic Energy and the Spring Constant Procedure Aim the launcher so that it will fire the ball straight up into the air. Measure the mass of the ball before setting the launcher up for a short range shot. Measure how much the ball has compressed the spring before shooting the ball into the air. Shoot the ball and measure its maximum height three times. Any value that clearly deviates from the other values, disregard it and retake that data point. Mass of the ball: 10g Spring Compression for short range shot: 3cm Height (m) 0.48 0.49 0.485 76-24 Calculate (a) the average height of the ball and (b) the gravitational potential energy at the ball's highest point. (think about where you choose to take y = 0) F a) 0.485 mah = 0.01 kg x 9.8m/52 x 0.52m kx X mv b) 0.010.48x9.8 = 0.04704 Calculate (a) the launch speed of the ball and (b) the spring constant of the launcher. a) V. = 3.285 m/s 3.1924 b) 1/3.24 0.04704 = 0.4 (9.8) +0.03) + = 110.03) 0.04998 = K(0.03) 0.00996 K(Q03) , I w CS Repeat the procedure for a medium range shot. Spring Compression for medium range shot: 6cm Height (m) 1.19 1.19 1-19 Calculate (a) the average height of the ball and (b) the gravitational potential energy at the ball's highest point. (think about where you choose to take y = 0) 9)1.19 b) 0.01X1.19x 9.8 = 0.11662 Calculate (a) the launch speed of the ball and (b) the spring constant of the launcher. a) 0.11662 = mu b) 0-11662=mgy + ky 483 V = 405 = 0.01 (9.8 m/s) (-0.06) + k ( -0.06) k=68.055 3 rs: io t 1 S t I static Calculate the % difference for your two spring constant values using the following formula. (larger value) - (smaller value) (larger value) % difference = 100 Part 2: Kinetic and Gravitational Potential Energy Procedure (Launch all balls using the medium range setting in Part 2.) Adjust the angle of the launcher to an unusual angle between 25 and 70. Measure the maximum vertical height of the ball. Do three trials so that you can calculate an average height. Any height that clearly deviates from the other values should be thrown out and retaken. Repeat for a second angle. Vertical Angle Height 1 Vertical Height 2 Average Vertical Vertical Height 3 Height 65 100 101 102 101 55 80 79.5 80 80 Calculate (a) the average height at each angle and (b) the gravitational potential energy at the ball's highest point for each launch angle. 4 () 1 1 1 Question 1. For each angle, use the launch speed calculated in Part 1 to predict what the speed of the ball is at its maximum height. Treat each of them as if they were the theoretical speed for the ball at their measured height. Question 2. For your larger average height, use conservation of energy to calculate the speed of the ball at that point. Clearly describe your reasoning and show clear calculations! Repeat for your smaller average height. Each is the experimental speed of the ball at their measured height. Question 3. Conservation of energy gives us the magnitude of the velocity vector, but not it's direction. What is the direction of the velocity vector at its peak height for the larger height? Is it the same for the smaller height? Question 4. Calculate the percent difference between the theoretical and experimental speeds of the ball at the maximum height. Repeat for your larger angle. % difference = |(theoretical value) - (experimental value)| (theoretical value) 100 Final Questions: To be answered on another sheet of paper after your analysis is com- pleted. Question 1. Should the medium range spring constant be the same as that found for the short range launch? Explain. Question 2. Briefly list the measured quantities that you used to determine the spring constant of the spring launcher. Rank them in terms of most accurate to least accurate and most precise to least precise. (I am not looking for a "correct" answer. I just want you to think and write your thoughts about it.) Question 3. Of the measured quantities you listed, which measurement do you believe had the largest effect on the calculated value of your spring constants? Explain. Question 4. Is the launch speed calculated in Part 1 a possible source of error in Part 2? Explain. 6