I've been traveling and it's been difficult to keep up with my Calculus class, everything has been running smoothly but now I got this assignment and I'm lost.

Here's the two assignments I'm working on, the one's I currently have already say "correct" under them, but I'm confused with all of it.

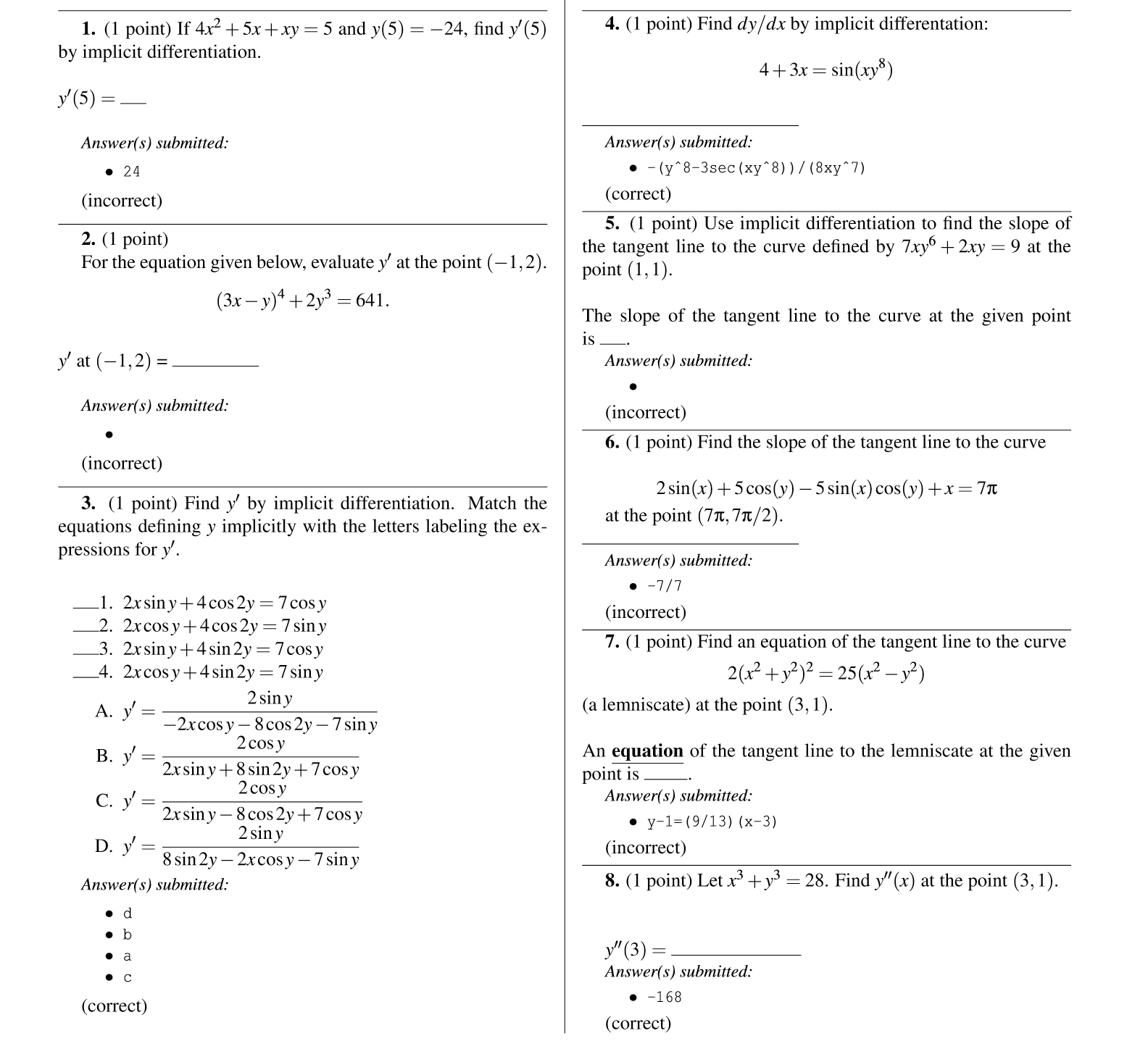
dy dx 1. (1 point) Suppose xy = l and a = 2. Find E when x = 1 dx _ dt _ Answer\") submitted: . (incorrect) 2. (1 point) Suppose that Jr : x(t} and y : y(t) are both functions oft. If 12 + y2 = 26, dx _ dy d=l h =1 (1 =5, ht ? and! wenx any walsdt dy _ dt _ Answes} submitted.- (incorrect) 3. (1 point) Suppose that x = x(t) and y = y(t} are both functions oft. If y2+xy3x:ll, d d anddf:4whenx=3andy:2,whatis dj? dx_ dt _ Answerr) submitted: (incorrect) 4. (1 point) A particle is moving along the curve y = 5\\/ 3x+ 1. As the particle passes through the point (5,20), its xcoordinate increases at a rate of 5 units per second. Find the rate of change of the distance from the particle to the origin at this instant. Answerr) submitted: (incorrect) 5. (1 point) The radius of a spherical balloon is increasing at a rate of 2 centimeters per minute. How fast is the volume changing when the radius is 14 centimeters? Note: The volume of a sphere is given by V = (4/3)1tr3. Rate of change of volume = (incorrect) 6. (1 point) Helium is pumped into a spherical balloon at a rate of 2 cu- bic feet per second. How fast is the radius increasing after 3 minutes? Note: The volume of a sphere is given by V = (4/3)Tcr3. Rate of change of radius (in feet per second) = Answerfs) submitted: (incorrect) 7. (1 point) A street light is at the top of a 17 ft tall pole. A woman 6 ft tall walks away from the pole with a speed of 6 ftlsec along a straight path. How fast is the tip of her shadow moving when she is 50 ft from the base of the pole? Note: You should draw a picture of a right triangle with the vertical side representing the pole, and the other end of the hy- potenuse representing the tip of the woman's shadow. Where does the woman t into this picture? Label her position as a variable, and label the tip of her shadow as another variable. You might like to use similar triangles to nd a relationship be- tween these two variables. Answerfs) submitted: (incorrect) 8. (1 point) Water is leaking out of an inverted conical tank at a rate of 7500.0 cubic centimeters per min at the same time that water is being pumped into the tank at a constant rate. The tank has height 15.0 meters and the diameter at the top is 4.0 meters. If the water level is rising at a rate of 18.0 centimeters per minute when the height of the water is 3.0 meters, nd the rate at which water is being pumped into the tank in cubic cen- timeters per minute. Note: Let \"R\" be the unknown rate at which water is being pumped in. Then you know that if V is volume of water, % = R 7500.0. Use geometry (similar triangles?) to nd the relationship between the height of the water and the volume of the water at any given time. Recall that the volume of a cone with base radius r and height h is given by %1l:r2h. Answerfs) submitted: (incorrect) 1. (1 point) If 41:2 + 5x+xy = 5 and y(5) = _24, nd y'(5) by implicit differentiation. f6)= Answes) submitted: 0 2 4 (incorrect) 2. (1 point) For the equation given below, evaluate y' at the point (1 , 2). (3Jty)4+2y3 : 641. y' at (1,?) = Answes) submitted: (incorrect) 3. (1 point) Find y' by implicit differentiation. Match the equations dening y implicitly with the letters labeling the ex- pressions for y'. _1. 2xsiny+4C052y=7cosy _2. 2xcosy+4cos2y27siny _3. lxsiny+4sin2y27cosy _4. 2xcosy+4si112y=7siny , 25iny A. y = . 21cosy8cosZy7smy , 2cosy B. y = + szmy+851n2y+7cosy , 2cosy C. y = , ZxSIny8c052y+7cosy D. y, = . 25iny - 831n2y2xc05y7smy Answes) submitted: I d o b O a o c (correct) 4. (1 point) Find dy/dx by implicit differentation: 4 + 3x = sin(xy8) Answerfs) submitted.- 0 -(y'8-3sec(xy'8) )/(8xy'7) (correct) 5. (1 point) Use implicit differentiation to nd the slope of the tangent line to the curve dened by 7xy6 +2.74); = 9 at the point (1,1). The slope of the tangent line to the curve at the given point is _. Answer( s ) submitted: (incorrect) 6. (1 point) Find the slope of the tangent line to the curve 2 sin(x) + 5 cos(y) 5 sin(x) cos(y) +x 2 71:: at the point (71:, 71t/2). Answetfs) submitted: 0 -7/7 (incorrect) 7. (1 point) Find an equation of the tangent line to the curve 2(x2 +322? = 25(x2 y2) (a lemniscate) at the point (3, 1). An equation of the tangent line to the lemniscate at the given point is Answeds) submitted: - Y'1=(9/13](x3) (incorrect) 8. (1 point) Let x3 +313 = 28. Find y\"(x) at the point (3, l). y\"(3)== Answeds) submitted.- 0 -168 (correct)