Java Programming. Just code needed, not answers to question. Please give me the code for this program all the way up to the step where it asks "repeat this for l = 1, 2, 3".

Thank you!

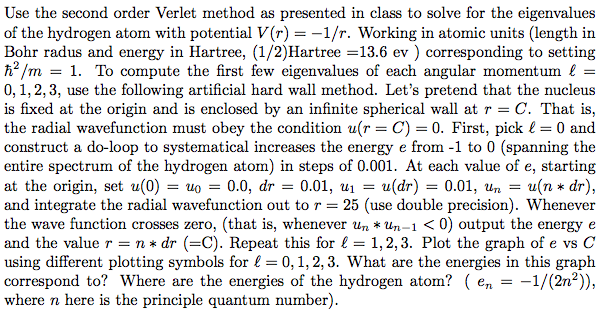
Use the second order Verlet method as presented in class to solve for the eigenvalues of the hydrogen atom with potential V(r) 1/r. Working in atomic units (length in Bohr radus and energy in Hartree, (1/2)Hartree 13.6 ev corresponding to setting h /m 1. To compute the first few eigenvalues of each angular momentum l 0, 1, 2, 3, use the following artificial hard wall method. Let's pretend that the nucleus is fixed at the origin and is enclosed by an infinite spherical wall at r C. That is, the radial wavefunction must obey the condition w(r C) 30. First, pick l 0 and construct a do-loop to systematical increases the energy e from -1 to 0 (spanning the entire spectrum of the hydrogen atom) in steps of 0.001. At each value of e, starting at the origin, set u(0) uo 0.0, dr 0.01, u u (dr) 0.01, un u(n dr), and integrate the radial wavefunction out to r 25 (use double precision). Whenever the wave function crosses zero, (that is, whenever un um-1 0) output the energy e and the value r n dr C). Repeat this for l 1, 2, 3. Plot the graph of e vs C using different plotting symbols for l 0, 1, 2, 3. What are the energies in this graph correspond to? Where are the energies of the hydrogen atom? en 1/(2n2)) where n here is the principle quantum number) Use the second order Verlet method as presented in class to solve for the eigenvalues of the hydrogen atom with potential V(r) 1/r. Working in atomic units (length in Bohr radus and energy in Hartree, (1/2)Hartree 13.6 ev corresponding to setting h /m 1. To compute the first few eigenvalues of each angular momentum l 0, 1, 2, 3, use the following artificial hard wall method. Let's pretend that the nucleus is fixed at the origin and is enclosed by an infinite spherical wall at r C. That is, the radial wavefunction must obey the condition w(r C) 30. First, pick l 0 and construct a do-loop to systematical increases the energy e from -1 to 0 (spanning the entire spectrum of the hydrogen atom) in steps of 0.001. At each value of e, starting at the origin, set u(0) uo 0.0, dr 0.01, u u (dr) 0.01, un u(n dr), and integrate the radial wavefunction out to r 25 (use double precision). Whenever the wave function crosses zero, (that is, whenever un um-1 0) output the energy e and the value r n dr C). Repeat this for l 1, 2, 3. Plot the graph of e vs C using different plotting symbols for l 0, 1, 2, 3. What are the energies in this graph correspond to? Where are the energies of the hydrogen atom? en 1/(2n2)) where n here is the principle quantum number)