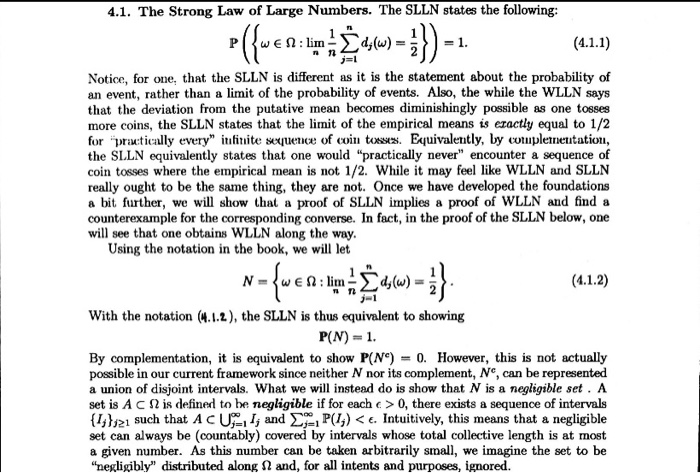
Let A, B be sets. We say that a function : A B is one-to-one or injective if f(1) = f(y) implies z = y. We say that A is an infinite set if there exists an injective function f: NA, where N denotes the natural numbers. We say that A is uncountably infinite if there exists an injective function f : [0,1) A. Given a set 2 and AC , we say that A is a proper subset of if AC and A The subtraction of B from A is the set A\B=An B. The symmetric difference of A and B is the set AAB= (A\B)U(B\A). The Aziom of Choice states that given any collection (Aalaez of sets, where I is any indexing set, one has Iloer Aa . Here, Iloez Aa denotes the product set of (Aa}a1. The product set is the set whose elements consist of any function f: I Uaer An such that f(a) A- Let A be a partially ordered collection of sets with partial ordering denoted by , i.e., is a relation on A that satisfies (Reflexivity) AA, for all A E A (Anti-symmetry) AB and BA implies A = B (Transitivity) AB and BC implies AC Zorn's Lemma states the following: If every chain, CC A, i.e., totally ordered sub- collection of A, i.e., for each A, B E C, one has AB or BA, has an upper bound, i.e., element UA such that AU, for all A e C, then A must have a maximal element, MEA, i.e., if MA, for some A A, then A = M. Recall that the topology of open intervals on R is the smallest subcollection, TC P(R), containing all open intervals (a, b) such that T is closed under the formation of finite intersections and arbitrary unions. 4.1. The Strong Law of Large Numbers. The SLLN states the following: >({wen m 46) = })=1 d; (w): 12 (4.1.1) Notice, for one, that the SLLN is different as it is the statement about the probability of an event, rather than a limit of the probability of events. Also, the while the WLLN says that the deviation from the putative mean becomes diminishingly possible as one tosses more coins, the SLLN states that the limit of the empirical means is exactly equal to 1/2 for practically every" infinite sequence of coin tosses. Equivalently, by complementation, the SLLN equivalently states that one would "practically never" encounter a sequence of coin tosses where the empirical mean is not 1/2. While it may feel like WLLN and SLLN really ought to be the same thing, they are not. Once we have developed the foundations a bit further, we will show that a proof of SLLN implies a proof of WLLN and find a counterexample for the corresponding converse. In fact, in the proof of the SLLN below, one will see that one obtains WLLN along the way. Using the notation in the book, we will let N = {WER: 4-}} (d, (w) (4.1.2) With the notation (4.1.2), the SLLN is thus equivalent to showing P(N) = 1. By complementation, it is equivalent to show P(N) = 0. However, this is not actually possible in our current framework since neither N nor its complement, N, can be represented a union of disjoint intervals. What we will instead do is show that N is a negligible set. A set is AC 2 is defined to be negligible if for each e > 0, there exists a sequence of intervals (21 such that A CUI, and , P(1)