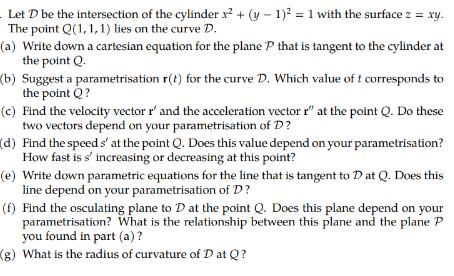
Let D be the intersection of the cylinder x + (y - 1) = 1 with the surface z = xy. The point Q(1,1,1) lies on the curve D. (a) Write down a cartesian equation for the plane P that is tangent to the cylinder at the point Q. (b) Suggest a parametrisation r(t) for the curve D. Which value of t corresponds to the point Q? (c) Find the velocity vector r' and the acceleration vector r" at the point Q. Do these two vectors depend on your parametrisation of D? (d) Find the speed s' at the point Q. Does this value depend on your parametrisation? How fast is s' increasing or decreasing at this point? (e) Write down parametric equations for the line that is tangent to D at Q. Does this line depend on your parametrisation of D? (f) Find the osculating plane to D at the point Q. Does this plane depend on your parametrisation? What is the relationship between this plane and the plane P you found in part (a)? (g) What is the radius of curvature of D at Q?