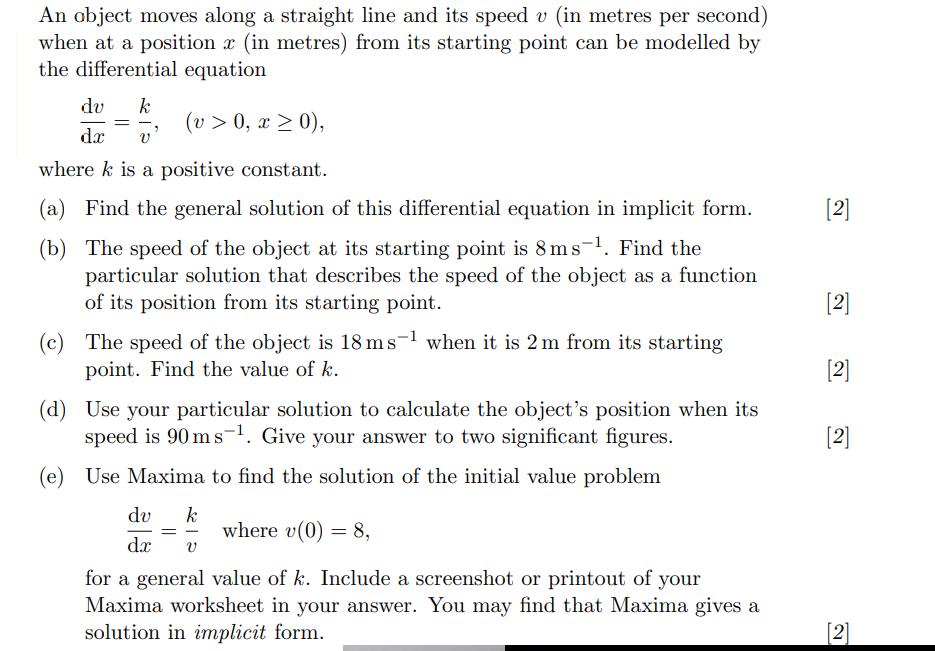
An object moves along a straight line and its speed v (in metres per second) when at a position x (in metres) from its starting point can be modelled by the differential equation dv k = - (v> 0, x > 0), dx where k is a positive constant. Find the general solution of this differential equation in implicit form. (b) The speed of the object at its starting point is 8 ms-. Find the particular solution that describes the speed of the object as a function of its position from its starting point. (c) The speed of the object is 18 ms - when it is 2 m from its starting point. Find the value of k. (d) Use your particular solution to calculate the object's position when its speed is 90 ms-. Give your answer to two significant figures. (e) Use Maxima to find the solution of the initial value problem dv k dx V where v(0) = 8, for a general value of k. Include a screenshot or printout of your Maxima worksheet in your answer. You may find that Maxima gives a solution in implicit form. = [2] [2] [2] [2] [2] An object moves along a straight line and its speed v (in metres per second) when at a position x (in metres) from its starting point can be modelled by the differential equation dv k = - (v> 0, x > 0), dx where k is a positive constant. Find the general solution of this differential equation in implicit form. (b) The speed of the object at its starting point is 8 ms-. Find the particular solution that describes the speed of the object as a function of its position from its starting point. (c) The speed of the object is 18 ms - when it is 2 m from its starting point. Find the value of k. (d) Use your particular solution to calculate the object's position when its speed is 90 ms-. Give your answer to two significant figures. (e) Use Maxima to find the solution of the initial value problem dv k dx V where v(0) = 8, for a general value of k. Include a screenshot or printout of your Maxima worksheet in your answer. You may find that Maxima gives a solution in implicit form. = [2] [2] [2] [2] [2]