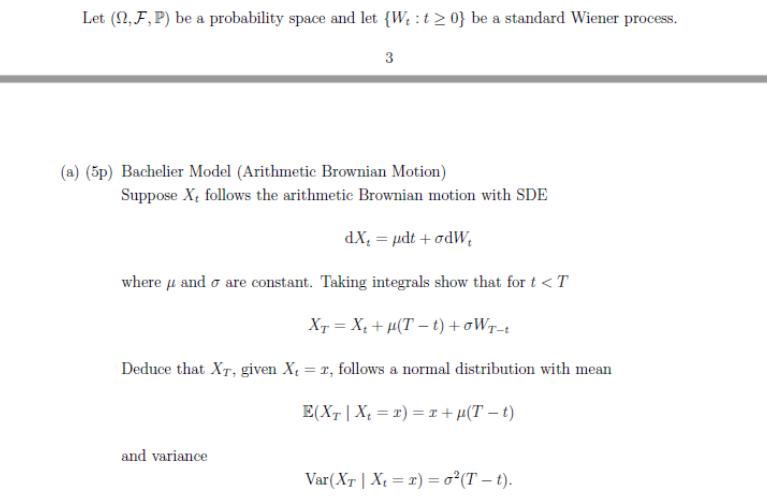
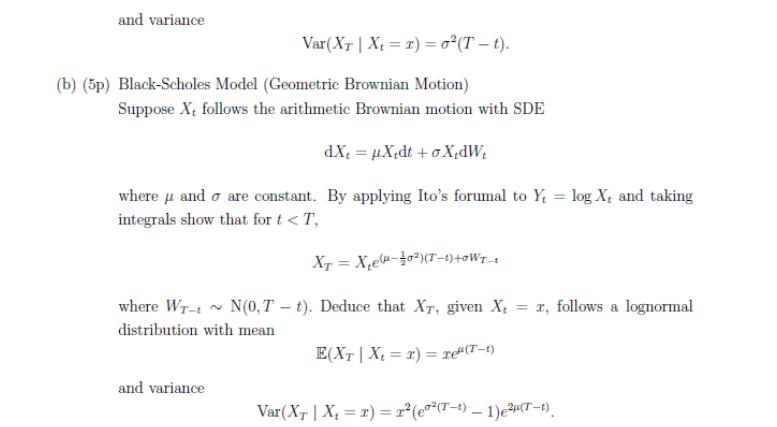
Let (,F,P) be a probability space and let (We:t>0} be a standard Wiener process. 3 (a) (5p) Bachelier Model (Arithmetic Brownian Motion) Suppose X, follows the arithmetic Brownian motion with SDE dX = = dt + odW where and o are constant. Taking integrals show that for t < T XT = X + (T-1) +OWT-t Deduce that XT, given X, = r, follows a normal distribution with mean E(XT | X = 1) = x+(T-t) and variance Var (XT | X = x)=0(T-t). and variance Var(XT | X = 2) = o (T-t). (b) (5p) Black-Scholes Model (Geometric Brownian Motion) Suppose X, follows the arithmetic Brownian motion with SDE dX = Xedt + o XdW where and are constant. By applying Ito's forumal to Y, = log X, and taking integrals show that for t < T, XT = Xe-0) (T-1)+oWT-1 where WT-t~ N(0,T-t). Deduce that Xr, given X = x, follows a lognormal distribution with mean E(XT | X = 1) = re(T-1) and variance Var (XT | X=2) = 1 (e(T-1)-1)e(T-t). (c) (5p) Generalized Geometric Brownian Motion Suppose X, follows the arithmetic Brownian motion with SDE dX = Xdt + oXdW where and are time dependent. By applying Ito's forumal to Y = log X, and taking integrals show that for t