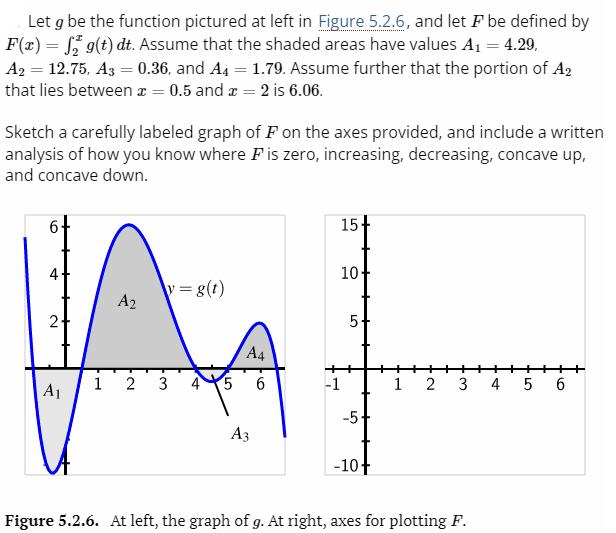
Let g be the function pictured at left in Figure 5.2.6, and let F be defined by F(x) = g(t) dt. Assume that the shaded areas have values A = 4.29, A2 = 12.75, A3 = 0.36, and A4 = 1.79. Assume further that the portion of A2 that lies between x = 0.5 and x = 2 is 6.06. Sketch a carefully labeled graph of F on the axes provided, and include a written analysis of how you know where F is zero, increasing, decreasing, concave up, and concave down. 6 4 2+ A U A y = g(t) A4 1 2 3 4 5 6 A3 -1 15 10+ 5- -5- -10+ 1 2 3 Figure 5.2.6. At left, the graph of g. At right, axes for plotting F. 4 5 6 5. The tide removes sand from the beach at a small ocean park at a rate modeled by the function R(t) = 2 + 5 sin 4t 25 A pumping station adds sand to the beach at rate modeled by the function 15t 1 + 3t S(t) = Both R(t) and S(t) are measured in cubic yards of sand per hour, t is measured in hours, and the valid times are 0 < t < 6. At time t = 0, the beach holds 2500 cubic yards of sand. a. What definite integral measures how much sand the tide will remove during the time period 0 < t < 6? Why? b. Write an expression for Y (), the total number of cubic yards of sand on the beach at time . Carefully explain your thinking and reasoning. c. At what instantaneous rate is the total number of cubic yards of sand on the beach at time t = 4 changing? d. Over the time interval 0 < t < 6, at what time t is the amount of sand on the beach least? What is this minimum value? Explain and justify your answers fully.