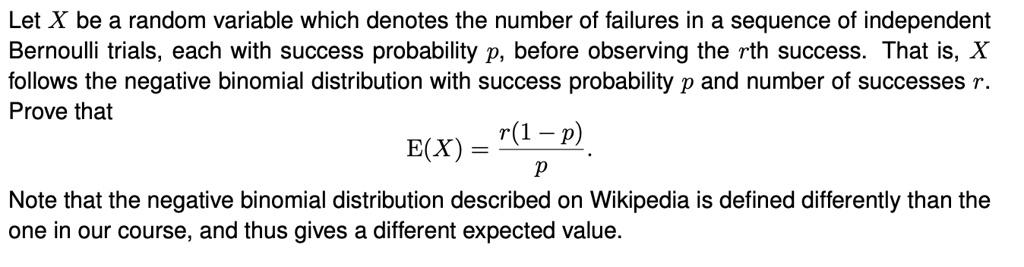
Let X be a random variable which denotes the number of failures in a sequence of independent Bernoulli trials, each with success probability p, before observing the rth success. That is, X follows the negative binomial distribution with success probability p and number of successes r. Prove that r(1 p) E(X)= Note that the negative binomial distribution described on Wikipedia is defined differently than the one in our course, and thus gives a different expected value.