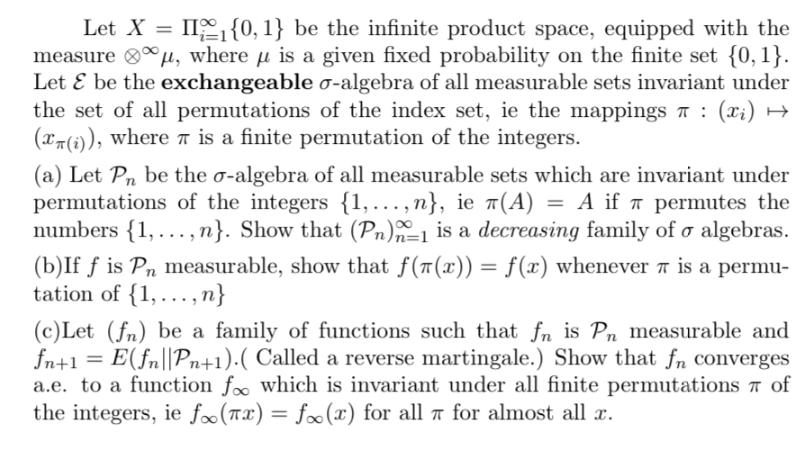
Let X = II{0, 1} be the infinite product space, equipped with the measure , where is a given fixed probability on the finite set {0,1}. Let & be the exchangeable -algebra of all measurable sets invariant under the set of all permutations of the index set, ie the mappings : (xi) (x(i)), where is a finite permutation of the integers. (a) Let Pn be the o-algebra of all measurable sets which are invariant under permutations of the integers {1,..., n}, ie (A) = A if 7 permutes the numbers {1,...,n}. Show that (Pn)=1 is a decreasing family of algebras. (b) If f is Pn measurable, show that f((x)) = f(x) whenever is a permu- tation of {1,..., n} (c) Let (fn) be a family of functions such that fn is Pn measurable and fn+1 = E(fn||Pn+1). ( Called a reverse martingale.) Show that fn converges a.e. to a function fo which is invariant under all finite permutations of the integers, ie f(Tx) = f(x) for all 7 for almost all x.