Linear Algebra: Solving second order differential equations

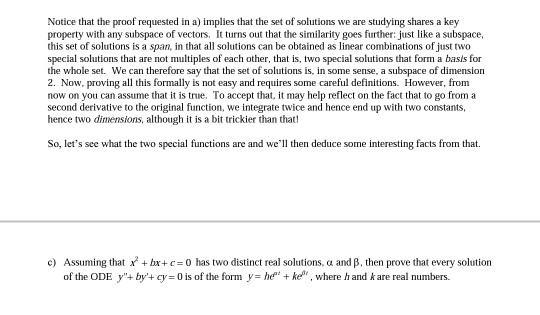
Notice that the proof requested in a) implies that the set of solutions we are studying shares a key property with any subspace of vectors. It turns out that the similarity goes further: just like a subspace, this set of solutions is a span, in that all solutions can be obtained as linear combinations of just two special solutions that are not multiples of each other, that is, two special solutions that form a basts for the whole set. We can therefore say that the set of solutions is, in some sense, a subspace of dimension 2. Now. proving all this formally is not easy and requires some careful definitions. However, from now on you can assume that it is true. To accept that, it may help reflect on the fact that to go from a second derivative to the original function, we integrate twice and hence end up with two constants, hence two dimensions, although is a bit trickier than that! So, let's see what the two special functions are and we'l then deduce some interesting facts from that. Assuming that x2 + hx+ c of the ODE y c) 0 has two distinct real solutions, and , then prove that every solution by'+ cy 0 is of the form y- he" +ke', where h and kare real numbers. d) Use the previous information to find the general solution of the ODE y" y-12y 0 e) Check that if + bx+ c has a unique real root a, so that it can be written as (x-a), then y-t is a solution to the ODE y"4 by't cy= 0 . Keep in mind that in this case =-(you may want to check that) 1) Explain why it is true that if a is the unique real solution of x+bx+ c0, then every solution of the ODE y"4 by't cy:0 is of the form y; hea, + kre" , where h and k are real numbers. g) Use what you found above to find the general solution of the ODE y"4 6y99y 0 h) Consider the third order ODE y"4 by"4 g4 dy:0 . and suppose that x, x, x, are three distinct, real solutions of the equation x+bcx+d 0. On the basis on what you have learned in the previous questions, make a reasonable conjecture about the form of the general solution of this ODE. Notice that the proof requested in a) implies that the set of solutions we are studying shares a key property with any subspace of vectors. It turns out that the similarity goes further: just like a subspace, this set of solutions is a span, in that all solutions can be obtained as linear combinations of just two special solutions that are not multiples of each other, that is, two special solutions that form a basts for the whole set. We can therefore say that the set of solutions is, in some sense, a subspace of dimension 2. Now. proving all this formally is not easy and requires some careful definitions. However, from now on you can assume that it is true. To accept that, it may help reflect on the fact that to go from a second derivative to the original function, we integrate twice and hence end up with two constants, hence two dimensions, although is a bit trickier than that! So, let's see what the two special functions are and we'l then deduce some interesting facts from that. Assuming that x2 + hx+ c of the ODE y c) 0 has two distinct real solutions, and , then prove that every solution by'+ cy 0 is of the form y- he" +ke', where h and kare real numbers. d) Use the previous information to find the general solution of the ODE y" y-12y 0 e) Check that if + bx+ c has a unique real root a, so that it can be written as (x-a), then y-t is a solution to the ODE y"4 by't cy= 0 . Keep in mind that in this case =-(you may want to check that) 1) Explain why it is true that if a is the unique real solution of x+bx+ c0, then every solution of the ODE y"4 by't cy:0 is of the form y; hea, + kre" , where h and k are real numbers. g) Use what you found above to find the general solution of the ODE y"4 6y99y 0 h) Consider the third order ODE y"4 by"4 g4 dy:0 . and suppose that x, x, x, are three distinct, real solutions of the equation x+bcx+d 0. On the basis on what you have learned in the previous questions, make a reasonable conjecture about the form of the general solution of this ODE